- Research Article
- Open access
- Published:
Common Fixed Point Theorems for a Finite Family of Discontinuous and Noncommutative Maps
Fixed Point Theory and Applications volume 2011, Article number: 847170 (2011)
Abstract
We study common fixed point theorems for a finite family of discontinuous and noncommutative single-valued functions defined in complete metric spaces. We also study a common fixed point theorem for two multivalued self-mappings and a stationary point theorem in complete metric spaces. Throughout this paper, we establish common fixed point theorems without commuting and continuity assumptions. In contrast, commuting or continuity assumptions are often assumed in common fixed point theorems. We also give examples to show our results. Results in this paper except those that generalized Banach contraction principle and those improve and generalize recent results in fixed point theorem are original and different from any existence result in the literature. The results in this paper will have some applications in nonlinear analysis and fixed point theory.
1. Introduction and Preliminaries
Let  be a metric space and
be a metric space and  be a multivalued map. We say that
be a multivalued map. We say that  is a stationary point of
is a stationary point of  if
if  . The existence theorem of stationary point was first considered by Dancs et al. [1]. If
. The existence theorem of stationary point was first considered by Dancs et al. [1]. If  is a self-mapping (multivalued or single valued) defined on
is a self-mapping (multivalued or single valued) defined on  , we denote
, we denote  the collection of all the fixed points of
the collection of all the fixed points of  . In this paper,we need the following definitions.
. In this paper,we need the following definitions.
Definition 1.1.
A function  is called
is called
(i)contraction if there exists  such that
such that

(ii)kannan if there exists  such that
such that

(iii)quasicontractive if there is a constant  such that
such that

where  .
.
(iv)weakly contractive if there exists a lower semicontinuous and nondecreasing function  with
with  if and only if
if and only if  such that
such that

It is known that every contraction and every Kannan mapping has a unique fixed point in complete metric spaces Banach [2], Kannan [3] and every quasicontractive mapping has a unique fixed point in Banach spaces Ćirić [4], Rhoades [5]. In 2001, Rhoades [6] proved that every weakly contractive mapping has a unique fixed point in a complete metric space. Let  and
and  be self-maps defined on
be self-maps defined on  ; the following inequality was considered in the study of common fixed points theorems Rhoades [5], Chang [7]:
; the following inequality was considered in the study of common fixed points theorems Rhoades [5], Chang [7]:

for some constant  and function
and function  .
.
If  and
and  satisfy the inequality (1.5) with
satisfy the inequality (1.5) with

then  and
and  are said to be a couple of quasicontractive mappings which is studied by Rhoades [5]. Chang [7] prove that every couple of quasicontractive mappings has a unique common fixed point in Banach spaces. Recently, Zhang and Song [8] proved a common fixed point theorem in complete metric spaces under the following assumption:
are said to be a couple of quasicontractive mappings which is studied by Rhoades [5]. Chang [7] prove that every couple of quasicontractive mappings has a unique common fixed point in Banach spaces. Recently, Zhang and Song [8] proved a common fixed point theorem in complete metric spaces under the following assumption:

where  .
.
The result of Zhang and Song [8] generalized the results in [2, 3, 5, 6]. Motivated by Chang [7], Zhang and Song [8], it is natural to ask whether there is a common fixed point of  and
and  in
in  satisfy inequality (1.5) with
satisfy inequality (1.5) with  . In this paper, we give a positive answer to this question in complete metric spaces.
. In this paper, we give a positive answer to this question in complete metric spaces.
Let  be a finite family of self-mappings on
be a finite family of self-mappings on  . If there is a nondecreasing, lower semicontinuous function
. If there is a nondecreasing, lower semicontinuous function  with
with  if and only if
if and only if  such that for every
such that for every  ,
,

where  , for all
, for all  , and
, and

Here,  is the identity map defined on
is the identity map defined on  and
and  . We show that
. We show that  have a unique common fixed point if
have a unique common fixed point if  is complete. As a special case of this result, we give a common fixed point theorem in complete metric spaces under the assumption that inequality (1.5) holds with
is complete. As a special case of this result, we give a common fixed point theorem in complete metric spaces under the assumption that inequality (1.5) holds with  . One of our results generalized Banach contraction principle, an example is given (Example 2.12) to show that the maps
. One of our results generalized Banach contraction principle, an example is given (Example 2.12) to show that the maps  above need not to be continuous. The assumption of continuity is often used in the existence theorems of fixed points [6, 9–14]. We also give an example to show that the family
above need not to be continuous. The assumption of continuity is often used in the existence theorems of fixed points [6, 9–14]. We also give an example to show that the family  above is not necessary to be commuting, and in contrast that the commutativity assumption is often used in the existence theorems of common fixed points [9, 10, 13, 15, 16]. Finally, we generalize some of our results to the case of multivalued maps.
above is not necessary to be commuting, and in contrast that the commutativity assumption is often used in the existence theorems of common fixed points [9, 10, 13, 15, 16]. Finally, we generalize some of our results to the case of multivalued maps.
Let  be multivalued maps satisfy
be multivalued maps satisfy

for some  (where
(where  denotes the Hausdorff metric). In fact, under the hypothesis that inequality (1.10) holds, we can show that
denotes the Hausdorff metric). In fact, under the hypothesis that inequality (1.10) holds, we can show that  and
and  for all
for all  if
if  and
and  have nonempty closed bounded values. Further we give a new stationary point theorem in complete metric spaces and illustrate with examples (Examples 3.4 and 3.8).
have nonempty closed bounded values. Further we give a new stationary point theorem in complete metric spaces and illustrate with examples (Examples 3.4 and 3.8).
2. Fixed Point Theorems
Throughout this paper, let  be a complete metric space and let
be a complete metric space and let  be the set of all positive integers. In this section, all the self-maps on
be the set of all positive integers. In this section, all the self-maps on  are single valued. The following theorem is the main result in this section.
are single valued. The following theorem is the main result in this section.
Theorem 2.1.
Let  be a finite family of self-mappings on
be a finite family of self-mappings on  . If there is a nondecreasing, lower semicontinuous function
. If there is a nondecreasing, lower semicontinuous function  with
with  if and only if
if and only if  such that for every
such that for every  ,
,

where

for all  , and
, and

 is the identity map defined on
is the identity map defined on  and
and  .
.
Then,  have a unique common fixed point.
have a unique common fixed point.
Proof.
For any fixed  , take
, take

Continuing in this way, we obtain by induction a sequence  in
in  such that
such that  , whenever
, whenever  with
with  and
and  . Then, if
. Then, if  , we have
, we have

If  for some
for some  , then
, then

Therefore  is a decreasing and bounded below sequence,and there exists
is a decreasing and bounded below sequence,and there exists  such that
such that  . Since
. Since  is lower semicontinuous,
is lower semicontinuous,  . Taking upper limits as
. Taking upper limits as  on two sides of the following inequality
on two sides of the following inequality

we have

Then,  and, hence,
and, hence,  .
.
 is a Cauchy sequence in
is a Cauchy sequence in  . Indeed, let
. Indeed, let  ,
,  . Then
. Then  is a decreasing sequence. If
is a decreasing sequence. If  , we are done. Suppose that
, we are done. Suppose that  , choose
, choose  small enough and select
small enough and select  such that
such that

By the definition of  , there exist
, there exist  such that
such that

Since  , for all
, for all  . Replace
. Replace  and
and  if necessary, we may assume that
if necessary, we may assume that  ,
,  and
and

Hence,

Then,

We consider the following two cases:
(i)
(ii) .
.
If  , we have
, we have

Then,  . Since
. Since  is arbitrary small positive number, if we take
is arbitrary small positive number, if we take  . Then,
. Then,

This yields a contradiction.
If  , we have
, we have

Then  . This also yields a contradiction.
. This also yields a contradiction.
Therefore,  and
and  is a Cauchy sequence in
is a Cauchy sequence in  . Since
. Since  is complete,
is complete,  converges to a point in
converges to a point in  , say
, say  .
.
In order to show that  is the unique common fixed point of
is the unique common fixed point of  . We first claim that
. We first claim that  , for all
, for all  .
.
Indeed, for each  ,
,

We consider the following three cases:
(i) ,
,
(ii) ,
,
(iii) .
.
If  , then
, then

If  , then
, then

If  , then
, then

Continuing in this process, we show that  . By the same argument as in the case above, we see that
. By the same argument as in the case above, we see that  .
.
Then, we see that  , for all
, for all  . Next, we claim that
. Next, we claim that  is the unique fixed point of
is the unique fixed point of  . Indeed, for any
. Indeed, for any  , we have
, we have

Then,  and
and  . Therefore,
. Therefore,  is the unique fixed point of
is the unique fixed point of  and we complete the proof.
and we complete the proof.
Remark 2.2.
-
(a)
The sequence
 approaching to the unique common fixed point in Theorem 2.1 is different from those in [8, 11, 12, 16–19].
approaching to the unique common fixed point in Theorem 2.1 is different from those in [8, 11, 12, 16–19]. -
(b)
The finite family
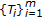 of self-mappings in Theorem 2.1 is neither commuting nor continuous, which are often assumed in common fixed point theorems, see [6, 9–16]. In fact, the commuting and continuity assumptions are not needed throughout this paper and we will give examples (Examples 2.12–2.15) to show this fact.
of self-mappings in Theorem 2.1 is neither commuting nor continuous, which are often assumed in common fixed point theorems, see [6, 9–16]. In fact, the commuting and continuity assumptions are not needed throughout this paper and we will give examples (Examples 2.12–2.15) to show this fact.
As special cases of Theorem 2.1, we have the following theorems and corollaries.
Theorem 2.3.
Let  , be self-mappings on
, be self-mappings on  . If there is a nondecreasing, lower semicontinuous function
. If there is a nondecreasing, lower semicontinuous function  with
with  if and only if
if and only if  such that
such that

for all  . Then
. Then  and
and  have a unique common fixed point.
have a unique common fixed point.
Proof.
Take  ,
,  and
and  in Theorem 2.1, then Theorem 2.3 follows from Theorem 2.1.
in Theorem 2.1, then Theorem 2.3 follows from Theorem 2.1.
Corollary 2.4.
Let  be self-mappings on
be self-mappings on  . If there is a nondecreasing, lower semicontinuous function
. If there is a nondecreasing, lower semicontinuous function  with
with  if and only if
if and only if  such that
such that

Then  and
and  have a unique common fixed point.
have a unique common fixed point.
Corollary 2.5.
Let  be a self-mapping on
be a self-mapping on  . If there is a nondecreasing, lower semicontinuous function
. If there is a nondecreasing, lower semicontinuous function  with
with  if and only if
if and only if  such that
such that

Then  has a unique fixed point.
has a unique fixed point.
Proof.
Take  in Theorem 2.3, then Corollary 2.4 follows from Corollary 2.4.
in Theorem 2.3, then Corollary 2.4 follows from Corollary 2.4.
Remark 2.6.
-
(a)
Since
 for all
for all  implies
implies  (2.25)
(2.25)
Corollary 2.5 generalizes Theorem 1 in Rhoades [6].
-
(b)
Corollary 2.4 is equivalent to Corollary 2.5.
Proof.
It suffices to show that  in Corollary 2.4. Indeed, for each
in Corollary 2.4. Indeed, for each  , there exists
, there exists  such that
such that  . By the hypothesis in Corollary 2.4,
. By the hypothesis in Corollary 2.4,  and we complete the proof.
and we complete the proof.
-
(c)
In Theorem 2.3, the map
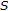 is not necessary equal
is not necessary equal 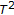 , see Example 2.14. In fact, the maps
, see Example 2.14. In fact, the maps  and
and  in Theorem 2.3 are not necessary to be commuting, see Example 2.15.
in Theorem 2.3 are not necessary to be commuting, see Example 2.15.
Theorem 2.7.
Let  be a finite family of self-mappings on
be a finite family of self-mappings on  . If there exists
. If there exists  such that for every
such that for every 

where

and  is the identity map defined on
is the identity map defined on  and
and  .
.
Then  have a unique common fixed point.
have a unique common fixed point.
Proof.
Take  for all
for all  , then Theorem 2.7 follows from Theorem 2.1.
, then Theorem 2.7 follows from Theorem 2.1.
Theorem 2.8.
Let  be self-mappings on
be self-mappings on  . If there exists
. If there exists  such that
such that

for all  . Then
. Then  and
and  have unique common fixed point.
have unique common fixed point.
Proof.
Take,  ,
,  and
and  in Theorem 2.7, then Theorem 2.8 follows from Theorem 2.7.
in Theorem 2.7, then Theorem 2.8 follows from Theorem 2.7.
Corollary 2.9.
Let  be self-mappings on
be self-mappings on  and if there exists
and if there exists  such that
such that

Then,  and
and  have a unique common fixed point.
have a unique common fixed point.
Corollary 2.10.
Let  be a self-map on
be a self-map on  and if there exists
and if there exists  such that
such that

Then  has a unique fixed point.
has a unique fixed point.
Proof.
Take  in Corollary 2.9, then Corollary 2.10 follows from Corollary 2.9.
in Corollary 2.9, then Corollary 2.10 follows from Corollary 2.9.
Remark 2.11.
-
(i)
If
 is contractive, then there exists
is contractive, then there exists 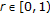 such that
such that  for all
for all  , but the converse is not true. It is obvious that Corollary 2.9 is a special case of Corollaries 2.4 and 2.10 is a generalization of Banach contraction principle. Further we see that Corollary 2.9 is equivalent to Corollary 2.10 by the same argument as in Remark 2.6.
, but the converse is not true. It is obvious that Corollary 2.9 is a special case of Corollaries 2.4 and 2.10 is a generalization of Banach contraction principle. Further we see that Corollary 2.9 is equivalent to Corollary 2.10 by the same argument as in Remark 2.6. -
(ii)
A map
 satisfies
satisfies  for all
for all 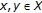 and for some
and for some  is neither continuous nor nonexpansive. We give an example (Example 2.12) to show this fact.
is neither continuous nor nonexpansive. We give an example (Example 2.12) to show this fact.
Example 2.12.
Let  be defined by
be defined by

Then,  for all
for all  and
and  is not continuous.
is not continuous.
Proof.
We consider the following three cases:
(i) ,
,
(ii) ,
,
(iii) .
.
If  ,
,  , then
, then

If  ,
,  , then
, then

If  ,
,  , then
, then

and, hence  . It is obvious that
. It is obvious that  is not continuous at
is not continuous at  but
but  for all
for all  .
.
Example 2.13.
Let  be the same as in Example 2.12. and take
be the same as in Example 2.12. and take  for all
for all  . Since
. Since  for all
for all  . By Example 2.12 and Corollary 2.10, we see that
. By Example 2.12 and Corollary 2.10, we see that  has a unique common fixed point. But for each
has a unique common fixed point. But for each  ,
,  is not continuous, the results in [13, 16] do not work in this example. Further it is obvious that the family
is not continuous, the results in [13, 16] do not work in this example. Further it is obvious that the family  have a unique common fixed point 0.
have a unique common fixed point 0.
Example 2.14.
Let  and define maps
and define maps  by
by  and
and  . Then
. Then  and we see that
and we see that  and
and  have a unique common fixed point.
have a unique common fixed point.
Proof.
It suffices to show that there exists  such that
such that

for all  .
.
We have to consider the following two cases:
(i) ,
,
(ii) .
.
If  , then
, then

If  , then
, then

If we take  , then by Theorem 2.8, we see that
, then by Theorem 2.8, we see that  and
and  have a unique common fixed point. In fact, 0 is the unique common fixed point of
have a unique common fixed point. In fact, 0 is the unique common fixed point of  and
and  .
.
By the same argument as in Example 2.14, we give the following example to show that the maps  and
and  in Theorem 2.8 are not necessary to be commuting.
in Theorem 2.8 are not necessary to be commuting.
Example 2.15.
Let  and define maps
and define maps  by
by  and
and  . Then
. Then  and
and  are not commuting and we see that
are not commuting and we see that  and
and  have a unique common fixed point.
have a unique common fixed point.
Proof.
It suffices to show that there exists  such that
such that

for all  .
.
We have to consider the following two cases:
(i) ,
,
(ii) .
.
If  , then
, then

If  , then
, then

If we take  , then by Theorem 2.8, we see
, then by Theorem 2.8, we see  and
and  have a unique common fixed point. In fact, 0 is the unique common fixed point of
have a unique common fixed point. In fact, 0 is the unique common fixed point of  and
and  .
.
3. A Common Fixed Point Theorem of Set-Valued Maps and a Stationary Point Theorem
In this section, we study a fixed point theorem and a stationary point theorem which generalize a fixed point theorem in Section 2.
In this section, let  be the class of all nonempty bounded closed subsets of
be the class of all nonempty bounded closed subsets of  and for
and for  , let
, let  be the Hausdorff metric of
be the Hausdorff metric of  and
and  and let
and let  for all
for all  .
.
Lemma 3.1 (see [20]).
For all  ,
,  and
and  , there exists
, there exists  such that
such that  .
.
Theorem 3.2.
Let  be multivalued maps. If there exists
be multivalued maps. If there exists  such that
such that

Then  and
and  for all
for all  .
.
Proof.
For any fixed  and
and  . Take
. Take  , and let
, and let  . By Lemma 3.1, we may choose
. By Lemma 3.1, we may choose  such that
such that  ,
,  such that
such that  ,
,  such that
such that  . Continuing in this process, we obtain by induction a sequence
. Continuing in this process, we obtain by induction a sequence  such that
such that

Therefore,

Therefore,  for all
for all  and
and

This shows that

and  is a Cauchy sequence. Since
is a Cauchy sequence. Since  is complete, there exists
is complete, there exists  such that
such that  . Since
. Since

and  ,
,  . Therefore
. Therefore  and
and  .
.
To complete the proof, it suffices to show the following four cases:
(i) and
and  for all
for all  ,
,
(ii) ,
,
(iii) for all
for all  ,
,
(iv) .
.
For any  ,
,

This shows that  and
and  . Further
. Further

and  .
.
For any  ,
,

This shows that  . Till now, we see that
. Till now, we see that  and
and  for all
for all  .
.
For any  ,
,

Hence  .
.
It remains to show that  .
.
Indeed, for any  ,
,

and  . Then
. Then  and
and  .
.
Remark 3.3.
-
(a)
If one of
 and
and  in Theorem 3.2 is single valued, then the set
in Theorem 3.2 is single valued, then the set 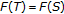 is singleton and the maps
is singleton and the maps 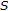 and
and  have a unique common fixed point in
have a unique common fixed point in 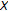 . Therefore, Theorem 3.2 is a generalization of Corollary 2.9, but Theorem 3.2 is not a generalization of Theorem 5 Nadler [20].
. Therefore, Theorem 3.2 is a generalization of Corollary 2.9, but Theorem 3.2 is not a generalization of Theorem 5 Nadler [20]. -
(b)
The sequence
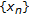 approaches to the common fixed point
approaches to the common fixed point  of
of  and
and  in Theorem 3.2 is different from those in [20–25].
in Theorem 3.2 is different from those in [20–25]. -
(c)
By Example 2.12, we see that both
 and
and  in Theorem 3.2 are neither to be upper semicontinuous nor to be lower semicontinuous (multivalued maps). Further the maps
in Theorem 3.2 are neither to be upper semicontinuous nor to be lower semicontinuous (multivalued maps). Further the maps  and
and 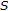 are not necessary to be commuting. We give an example below.
are not necessary to be commuting. We give an example below.
Example 3.4.
Let  and let maps
and let maps  be defined by
be defined by  and
and  . Then we see that
. Then we see that  and
and  have a unique common fixed point.
have a unique common fixed point.
Proof.
It suffices to show that there exists  such that
such that

We have to consider the following two cases:
(i)
(ii)
If  , we have
, we have

If  , we have
, we have

If we take  , then by Theorem 3.2, we see
, then by Theorem 3.2, we see  and
and  have a unique common fixed point. In fact, 0 is the unique common fixed point of
have a unique common fixed point. In fact, 0 is the unique common fixed point of  and
and  .
.
Corollary 3.5.
Let  be a multivalued map with nonempty compact values and
be a multivalued map with nonempty compact values and  such that
such that

Then  and
and  for all
for all  .
.
Similarly, we have the following existence theorem of stationary points.
Theorem 3.6.
Let  be a multivalued map,
be a multivalued map,  be a single valued function. If
be a single valued function. If  is a nondecreasing, lower semicontinuous function with
is a nondecreasing, lower semicontinuous function with  for all
for all  and
and  if and only if
if and only if  . Suppose that
. Suppose that

Then  has a unique stationary point, say
has a unique stationary point, say  . In fact,
. In fact,  and
and  .
.
Proof.
For any fixed  , let
, let  ,
,  ,
,  ,
,  . Continuing in this process, we obtain by induction a sequence
. Continuing in this process, we obtain by induction a sequence  such that
such that  and
and  . Since
. Since

Then,  is a decreasing and bounded below sequence, and hence there exist
is a decreasing and bounded below sequence, and hence there exist  such that
such that  . Since
. Since  is lower semicontinuous,
is lower semicontinuous,  . Taking upper limits as
. Taking upper limits as  on two sides of the following inequality
on two sides of the following inequality

we have

Then,  and hence
and hence  .
.
 is a Cauchy sequence in
is a Cauchy sequence in  . Indeed, let
. Indeed, let  . Then
. Then  is a decreasing sequence. If
is a decreasing sequence. If  , we are done. Suppose that
, we are done. Suppose that  , choose
, choose  small enough and select
small enough and select  such that
such that

By the definition of  , there exists
, there exists  such that
such that

Since  , for all
, for all  . Replace
. Replace  and
and  if necessary, we may assume that
if necessary, we may assume that  ,
,  and
and

Hence,

Then,

and  . Since
. Since  is arbitrary small positive number, if we take
is arbitrary small positive number, if we take  . Then
. Then

This yields a contradiction. Therefore  and
and  is a Cauchy sequence in
is a Cauchy sequence in  . By the completeness of
. By the completeness of  ,
,  converges to a point in
converges to a point in  , say
, say  .
.
Since,

It follows that  .
.
Further for all  ,
,

Then,  and
and  .
.
For all  ,
,

Then,  and hence
and hence  .
.
Remark 3.7.
-
(a)
The single valued map
 in Theorem 3.6 is not necessary to be continuous (see Example 2.12), but the continuity assumption is used in Theorem 3.2 [21, 22, 25] and Theorem 2.1 Ćirić and Ume [23]. We give an example to show that
in Theorem 3.6 is not necessary to be continuous (see Example 2.12), but the continuity assumption is used in Theorem 3.2 [21, 22, 25] and Theorem 2.1 Ćirić and Ume [23]. We give an example to show that  and
and 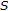 in Theorem 3.6 are not necessary to be commuting.
in Theorem 3.6 are not necessary to be commuting. -
(b)
Theorems 3.2 and 3.6 are different and Theorem 3.6 is also a generalization of Corollary 2.9.
Example 3.8.
Let  and let maps
and let maps  and
and  be defined by
be defined by  and
and  . Then we see that
. Then we see that  has a unique stationary point.
has a unique stationary point.
Proof.
It suffices to show that there exists  such that
such that

We have to consider the following two cases:
(i) ,
,
(ii) .
.
If  , we have
, we have

If  , we have
, we have

If we take  . Then by Theorem 3.6, we see
. Then by Theorem 3.6, we see  has a unique stationary point. In fact, 0 is the unique stationary point of
has a unique stationary point. In fact, 0 is the unique stationary point of  .
.
References
Dancs S, Hegedüs M, Medvegyev P: A general ordering and fixed-point principle in complete metric space. Acta Scientiarum Mathematicarum 1983,46(1–4):381–388.
Banach S: Sur les operations dans les ensembles abstraits et leur application aux equations integrales. Fundamenta Mathematican 1922, 3: 133–181.
Kannan R: Some results on fixed points—II. The American Mathematical Monthly 1969, 76: 405–408. 10.2307/2316437
Ćirić LB: A generalization of Banach's contraction principle. Proceedings of the American Mathematical Society 1974, 45: 267–273.
Rhoades BE: A comparison of various definitions of contractive mappings. Transactions of the American Mathematical Society 1977, 226: 257–290.
Rhoades BE: Some theorems on weakly contractive maps. Nonlinear Analysis. Theory, Methods & Applications 2001, 47: 2683–2693. 10.1016/S0362-546X(01)00388-1
Chang SS: Random fixed point theorem in probabilistic analysis. Nonlinear Analysis 1981,5(2):113–122. 10.1016/0362-546X(81)90037-7
Zhang Q, Song Y: Fixed point theory for generalized φ -weak contractions. Applied Mathematics Letters 2009,22(1):75–78. 10.1016/j.aml.2008.02.007
Al-Thagafi MA: Common fixed points and best approximation. Journal of Approximation Theory 1996,85(3):318–323. 10.1006/jath.1996.0045
Ilić D, Rakočević V: Common fixed points for maps on cone metric space. Journal of Mathematical Analysis and Applications 2008,341(2):876–882. 10.1016/j.jmaa.2007.10.065
Ishikawa S: Fixed points by a new iteration method. Proceedings of the American Mathematical Society 1974, 44: 147–150. 10.1090/S0002-9939-1974-0336469-5
Ishikawa S: Fixed points and iteration of a nonexpansive mapping in a Banach space. Proceedings of the American Mathematical Society 1976,59(1):65–71. 10.1090/S0002-9939-1976-0412909-X
Kaewcharoen A, Kirk WA: Nonexpansive mappings defined on unbounded domains. Fixed Point Theory and Applications 2006, 2006:-13.
Oliveira P: Two results on fixed points. Nonlinear Analysis. Theory, Methods & Applicationsand Methods 2001, 47: 2703–2717. 10.1016/S0362-546X(01)00390-X
Kuczumow T, Reich S, Shoikhet D: The existence and non-existence of common fixed points for commuting families of holomorphic mappings. Nonlinear Analysis. Theory, Methods & Applications 2001,43(1):45–59. 10.1016/S0362-546X(99)00175-3
Xiao J-Z, Zhu X-H: Common fixed point theorems on weakly contractive and nonexpansive mappings. Fixed Point Theory and Applications 2008, 2008:-8.
Kirk WA: On successive approximations for nonexpansive mappings in Banach spaces. Glasgow Mathematical Journal 1971, 12: 6–9. 10.1017/S0017089500001063
Pant RP: Common fixed point theorems for contractive maps. Journal of Mathematical Analysis and Applications 1998,226(1):251–258. 10.1006/jmaa.1998.6029
Shimizu T, Takahashi W: Strong convergence to common fixed points of families of nonexpansive mappings. Journal of Mathematical Analysis and Applications 1997,211(1):71–83. 10.1006/jmaa.1997.5398
Nadler, SB Jr.: Multi-valued contraction mappings. Pacific Journal of Mathematics 1969, 30: 475–488.
Ahmad A, Imdad M: Some common fixed point theorems for mappings and multi-valued mappings. Journal of Mathematical Analysis and Applications 1998,218(2):546–560. 10.1006/jmaa.1997.5738
Ahmed A, Khan AR: Some common fixed point theorems for non-self-hybrid contractions. Journal of Mathematical Analysis and Applications 1997,213(1):275–286. 10.1006/jmaa.1997.5537
Ćirić LB, Ume JS: Some common fixed point theorems for weakly compatible mappings. Journal of Mathematical Analysis and Applications 2006,314(2):488–499. 10.1016/j.jmaa.2005.04.007
Daffer PZ, Kaneko H: Fixed points of generalized contractive multi-valued mappings. Journal of Mathematical Analysis and Applications 1995,192(2):655–666. 10.1006/jmaa.1995.1194
Imdad M, Khan L: Fixed point theorems for a family of hybrid pairs of mappings in metrically convex spaces. Fixed Point Theory and Applications 2005, (3):281–294.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License (https://creativecommons.org/licenses/by/2.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
About this article
Cite this article
Lin, LJ., Wang, SY. Common Fixed Point Theorems for a Finite Family of Discontinuous and Noncommutative Maps. Fixed Point Theory Appl 2011, 847170 (2011). https://doi.org/10.1155/2011/847170
Received:
Accepted:
Published:
DOI: https://doi.org/10.1155/2011/847170
 approaching to the unique common fixed point in Theorem 2.1 is different from those in [
approaching to the unique common fixed point in Theorem 2.1 is different from those in [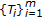 of self-mappings in Theorem 2.1 is neither commuting nor continuous, which are often assumed in common fixed point theorems, see [
of self-mappings in Theorem 2.1 is neither commuting nor continuous, which are often assumed in common fixed point theorems, see [ for all
for all  implies
implies 
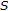 is not necessary equal
is not necessary equal 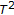 , see Example 2.14. In fact, the maps
, see Example 2.14. In fact, the maps  and
and  in Theorem 2.3 are not necessary to be commuting, see Example 2.15.
in Theorem 2.3 are not necessary to be commuting, see Example 2.15. is contractive, then there exists
is contractive, then there exists 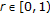 such that
such that  for all
for all  , but the converse is not true. It is obvious that Corollary 2.9 is a special case of Corollaries 2.4 and 2.10 is a generalization of Banach contraction principle. Further we see that Corollary 2.9 is equivalent to Corollary 2.10 by the same argument as in Remark 2.6.
, but the converse is not true. It is obvious that Corollary 2.9 is a special case of Corollaries 2.4 and 2.10 is a generalization of Banach contraction principle. Further we see that Corollary 2.9 is equivalent to Corollary 2.10 by the same argument as in Remark 2.6. satisfies
satisfies  for all
for all 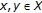 and for some
and for some  is neither continuous nor nonexpansive. We give an example (Example 2.12) to show this fact.
is neither continuous nor nonexpansive. We give an example (Example 2.12) to show this fact. and
and  in Theorem 3.2 is single valued, then the set
in Theorem 3.2 is single valued, then the set 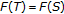 is singleton and the maps
is singleton and the maps 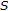 and
and  have a unique common fixed point in
have a unique common fixed point in 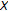 . Therefore, Theorem 3.2 is a generalization of Corollary 2.9, but Theorem 3.2 is not a generalization of Theorem 5 Nadler [
. Therefore, Theorem 3.2 is a generalization of Corollary 2.9, but Theorem 3.2 is not a generalization of Theorem 5 Nadler [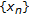 approaches to the common fixed point
approaches to the common fixed point  of
of  and
and  in Theorem 3.2 is different from those in [
in Theorem 3.2 is different from those in [ and
and  in Theorem 3.2 are neither to be upper semicontinuous nor to be lower semicontinuous (multivalued maps). Further the maps
in Theorem 3.2 are neither to be upper semicontinuous nor to be lower semicontinuous (multivalued maps). Further the maps  and
and 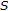 are not necessary to be commuting. We give an example below.
are not necessary to be commuting. We give an example below. in Theorem 3.6 is not necessary to be continuous (see Example 2.12), but the continuity assumption is used in Theorem 3.2 [
in Theorem 3.6 is not necessary to be continuous (see Example 2.12), but the continuity assumption is used in Theorem 3.2 [ and
and 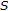 in Theorem 3.6 are not necessary to be commuting.
in Theorem 3.6 are not necessary to be commuting.