- Research Article
- Open access
- Published:
Viscosity approximation fixed points for nonexpansive and 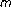 -accretive operators
-accretive operators
Fixed Point Theory and Applications volume 2006, Article number: 81325 (2006)
Abstract
Let  be a real reflexive Banach space, let
be a real reflexive Banach space, let  be a closed convex subset of
be a closed convex subset of  , and let
, and let  be an
be an  -accretive operator with a zero. Consider the iterative method that generates the sequence
-accretive operator with a zero. Consider the iterative method that generates the sequence  by the algorithm
by the algorithm  where
where  and
and  are two sequences satisfying certain conditions,
are two sequences satisfying certain conditions,  denotes the resolvent
denotes the resolvent  for
for  , and let
, and let  be a fixed contractive mapping. The strong convergence of the algorithm
be a fixed contractive mapping. The strong convergence of the algorithm  is proved assuming that
is proved assuming that  has a weakly continuous duality map.
has a weakly continuous duality map.
References
Barbu V: Nonlinear Semigroups and Differential Equations in Banach Spaces. Editura Academiei Republicii Socialiste România, Bucharest; Noordhoff, Leiden; 1976:352.
Browder FE: Convergence of approximants to fixed points of nonexpansive non-linear mappings in Banach spaces. Archive for Rational Mechanics and Analysis 1967,24(1):82–90.
Browder FE: Convergence theorems for sequences of nonlinear operators in Banach spaces. Mathematische Zeitschrift 1967,100(3):201–225. 10.1007/BF01109805
Lim T-C, Xu H-K: Fixed point theorems for asymptotically nonexpansive mappings. Nonlinear Analysis 1994,22(11):1345–1355. 10.1016/0362-546X(94)90116-3
Xu H-K: Iterative algorithms for nonlinear operators. Journal of the London Mathematical Society. Second Series 2002,66(1):240–256. 10.1112/S0024610702003332
Xu H-K: An iterative approach to quadratic optimization. Journal of Optimization Theory and Applications 2003,116(3):659–678. 10.1023/A:1023073621589
Xu H-K: Viscosity approximation methods for nonexpansive mappings. Journal of Mathematical Analysis and Applications 2004,298(1):279–291. 10.1016/j.jmaa.2004.04.059
Xu H-K: Strong convergence of an iterative method for nonexpansive and accretive operators. Journal of Mathematical Analysis and Applications 2006,314(2):631–643. 10.1016/j.jmaa.2005.04.082
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License (https://creativecommons.org/licenses/by/2.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
About this article
Cite this article
Chen, R., Zhu, Z. Viscosity approximation fixed points for nonexpansive and 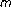 -accretive operators.
Fixed Point Theory Appl 2006, 81325 (2006). https://doi.org/10.1155/FPTA/2006/81325
-accretive operators.
Fixed Point Theory Appl 2006, 81325 (2006). https://doi.org/10.1155/FPTA/2006/81325
Received:
Accepted:
Published:
DOI: https://doi.org/10.1155/FPTA/2006/81325