- Research Article
- Open access
- Published:
Strong Convergence of Modified Halpern Iterations in CAT(0) Spaces
Fixed Point Theory and Applications volume 2011, Article number: 869458 (2011)
Abstract
Strong convergence theorems are established for the modified Halpern iterations of nonexpansive mappings in CAT(0) spaces. Our results extend and improve the recent ones announced by Kim and Xu (2005), Hu (2008), Song and Chen (2008), Saejung (2010), and many others.
1. Introduction
Let  be a nonempty subset of a metric space
be a nonempty subset of a metric space  . A mapping
. A mapping  is said to be nonexpansive if
is said to be nonexpansive if

A point  is called a fixed point of
is called a fixed point of  if
if  . We will denote by
. We will denote by  the set of fixed points of
the set of fixed points of  . In 1967, Halpern [1] introduced an explicit iterative scheme for a nonexpansive mapping
. In 1967, Halpern [1] introduced an explicit iterative scheme for a nonexpansive mapping  on a subset
on a subset  of a Hilbert space by taking any points
of a Hilbert space by taking any points  and defined the iterative sequence
and defined the iterative sequence  by
by

where  . He pointed out that the control conditions: (C1)
. He pointed out that the control conditions: (C1)  and (C2)
and (C2)  are necessary for the convergence of
are necessary for the convergence of  to a fixed point of
to a fixed point of  . Subsequently, many mathematicians worked on the Halpern iterations both in Hilbert and Banach spaces (see, e.g., [2–11] and the references therein). Among other things, Wittmann [7] proved strong convergence of the Halpern iteration under the control conditions (C1), (C2), and (C4)
. Subsequently, many mathematicians worked on the Halpern iterations both in Hilbert and Banach spaces (see, e.g., [2–11] and the references therein). Among other things, Wittmann [7] proved strong convergence of the Halpern iteration under the control conditions (C1), (C2), and (C4)  in a Hilbert space. In 2005, Kim and Xu [12] generalized Wittmann's result by introducing a modified Halpern iteration in a Banach space as follows. Let
in a Hilbert space. In 2005, Kim and Xu [12] generalized Wittmann's result by introducing a modified Halpern iteration in a Banach space as follows. Let  be a closed convex subset of a uniformly smooth Banach space
be a closed convex subset of a uniformly smooth Banach space  , and let
, and let  be a nonexpansive mapping. For any points
be a nonexpansive mapping. For any points  , the sequence
, the sequence  is defined by
is defined by

where  and
and  are sequences in
are sequences in  . They proved under the following control conditions:
. They proved under the following control conditions:

that the sequence  converges strongly to a fixed point of
converges strongly to a fixed point of  .
.
The purpose of this paper is to extend Kim-Xu's result to a special kind of metric spaces, namely, CAT(0) spaces. We also prove a strong convergence theorem for another kind of modified Halpern iteration defined by Hu [13] in this setting.
2. CAT(0) Spaces
A metric space  is a CAT(0) space if it is geodesically connected and if every geodesic triangle in
is a CAT(0) space if it is geodesically connected and if every geodesic triangle in  is at least as "thin" as its comparison triangle in the Euclidean plane. The precise definition is given below. It is well known that any complete, simply connected Riemannian manifold having nonpositive sectional curvature is a CAT(0) space. Other examples include Pre-Hilbert spaces (see [14]),
is at least as "thin" as its comparison triangle in the Euclidean plane. The precise definition is given below. It is well known that any complete, simply connected Riemannian manifold having nonpositive sectional curvature is a CAT(0) space. Other examples include Pre-Hilbert spaces (see [14]),  -trees (see [15]), Euclidean buildings (see [16]), the complex Hilbert ball with a hyperbolic metric (see [17]), and many others. For a thorough discussion of these spaces and of the fundamental role they play in geometry, we refer the reader to Bridson and Haefliger [14].
-trees (see [15]), Euclidean buildings (see [16]), the complex Hilbert ball with a hyperbolic metric (see [17]), and many others. For a thorough discussion of these spaces and of the fundamental role they play in geometry, we refer the reader to Bridson and Haefliger [14].
Fixed point theory in CAT(0) spaces was first studied by Kirk (see [18, 19]). He showed that every nonexpansive (single-valued) mapping defined on a bounded closed convex subset of a complete CAT(0) space always has a fixed point. Since then, the fixed point theory for single-valued and multivalued mappings in CAT(0) spaces has been rapidly developed, and many papers have appeared (see, e.g., [20–31] and the references therein). It is worth mentioning that fixed point theorems in CAT(0) spaces (specially in  -trees) can be applied to graph theory, biology, and computer science (see, e.g., [15, 32–35]).
-trees) can be applied to graph theory, biology, and computer science (see, e.g., [15, 32–35]).
Let  be a metric space. A geodesic path joining
be a metric space. A geodesic path joining  to
to  (or, more briefly, a geodesic from
(or, more briefly, a geodesic from  to
to  ) is a map
) is a map  from a closed interval
from a closed interval  to
to  such that
such that  and
and  for all
for all  . In particular,
. In particular,  is an isometry and
is an isometry and  . The image
. The image  of
of  is called a geodesic (or metric) segment joining
is called a geodesic (or metric) segment joining  and
and  . When it is unique, this geodesic segment is denoted by
. When it is unique, this geodesic segment is denoted by  . The space
. The space  is said to be a geodesic space if every two points of
is said to be a geodesic space if every two points of  are joined by a geodesic, and
are joined by a geodesic, and  is said to be uniquely geodesic if there is exactly one geodesic joining
is said to be uniquely geodesic if there is exactly one geodesic joining  and
and  for each
for each  . A subset
. A subset  is said to be convex if
is said to be convex if  includes every geodesic segment joining any two of its points.
includes every geodesic segment joining any two of its points.
A geodesic triangle
 in a geodesic metric space
in a geodesic metric space  consists of three points
consists of three points  in
in  (thevertices of
(thevertices of  ) and a geodesic segment between each pair of vertices (the edges of
) and a geodesic segment between each pair of vertices (the edges of  ). A comparison triangle for the geodesic triangle
). A comparison triangle for the geodesic triangle  in
in  is a triangle
is a triangle  in the Euclidean plane
in the Euclidean plane  such that
such that  for
for  .
.
A geodesic space is said to be a CAT(0) space if all geodesic triangles satisfy the following comparison axiom.
CAT(0): let  be a geodesic triangle in
be a geodesic triangle in  , and let
, and let  be a comparison triangle for
be a comparison triangle for  . Then,
. Then,  is said to satisfy the CAT(0) inequality if for all
is said to satisfy the CAT(0) inequality if for all  and all comparison points
and all comparison points  ,
,

Let  , and by Lemma 2.1 (iv) of [23] for each
, and by Lemma 2.1 (iv) of [23] for each  , there exists a unique point
, there exists a unique point  such that
such that

From now on, we will use the notation  for the unique point
for the unique point  satisfying (2.2). We now collect some elementary facts about CAT(0) spaces which will be used in the proofs of our main results.
satisfying (2.2). We now collect some elementary facts about CAT(0) spaces which will be used in the proofs of our main results.
Lemma 2.1.
Let  be a
be a  space. Then,
space. Then,
-
(i)
(see [23, Lemma 2.4]) for each
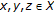 and
and  , one has
, one has 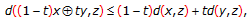 (23)
(23)
-
(ii)
(see [21]) for each
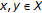 and
and 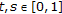 , one has
, one has 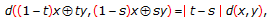 (24)
(24)
(iii) (see [19, Lemma 3]) for each  and
and  , one has
, one has

(iv) (see [23, Lemma 2.5]) for each  and
and  , one has
, one has

Recall that a continuous linear functional  on
on  , the Banach space of bounded real sequences, is called a Banach limit if
, the Banach space of bounded real sequences, is called a Banach limit if  and
and  for all
for all  .
.
Lemma 2.2 (see [8, Proposition 2]).
Let  be such that
be such that  for all Banach limits
for all Banach limits  and
and  . Then,
. Then,  .
.
Lemma 2.3 (see [28, Lemma 2.1]).
Let  be a closed convex subset of a complete
be a closed convex subset of a complete  space
space  , and let
, and let  be a nonexpansive mapping. Let
be a nonexpansive mapping. Let  be fixed. For each
be fixed. For each  , the mapping
, the mapping  defined by
defined by

has a unique fixed point  , that is,
, that is,

Lemma 2.4 (see [28, Lemma 2.2]).
Let  and
and  be as the preceding lemma. Then,
be as the preceding lemma. Then,  if and only if
if and only if  given by (2.8) remains bounded as
given by (2.8) remains bounded as  . In this case, the following statements hold:
. In this case, the following statements hold:
(1) converges to the unique fixed point
converges to the unique fixed point  of
of  which is nearest
which is nearest  ,
,
(2) for all Banach limits
for all Banach limits  and all bounded sequences
and all bounded sequences  with
with  .
.
Lemma 2.5 (see [10, Lemma 2.1]).
Let  be a sequence of nonnegative real numbers satisfying the condition
be a sequence of nonnegative real numbers satisfying the condition

where  and
and  are sequences of real numbers such that
are sequences of real numbers such that
(1) and
and  ,
,
-
(2)
either
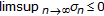 or
or  .
.
Then,  .
.
Let  and
and  be bounded sequences in a
be bounded sequences in a  space
space  , and let
, and let  be a sequence in
be a sequence in  with
with  . Suppose that
. Suppose that  for all
for all  and
and

Then,  .
.
3. Main Results
The following result is an analog of Theorem 1 of Kim and Xu [12]. They prove the theorem by using the concept of duality mapping, while we use the concept of Banach limit. We also observe that the condition  in [12, Theorem 1] is superfluous.
in [12, Theorem 1] is superfluous.
Theorem 3.1.
Let  be a nonempty closed convex subset of a complete
be a nonempty closed convex subset of a complete  space
space  , and let
, and let  be a nonexpansive mapping such that
be a nonexpansive mapping such that  . Given a point
. Given a point  and sequences
and sequences  and
and  in
in  , the following conditions are satisfied:
, the following conditions are satisfied:
(A1)  and
and  ,
,
(A2)  ,
,  and
and  .
.
Define a sequence  in
in  by
by  arbitrarily, and
arbitrarily, and

Then,  converges to a fixed point
converges to a fixed point  which is nearest
which is nearest  .
.
Proof.
For each  , we let
, we let  . We divide the proof into 3 steps. (i) We will show that
. We divide the proof into 3 steps. (i) We will show that  ,
,  , and
, and  are bounded sequences. (ii) We show that
are bounded sequences. (ii) We show that  . Finally, we show that (iii)
. Finally, we show that (iii)  converges to a fixed point
converges to a fixed point  which is nearest
which is nearest  .
.
-
(i)
As in the first part of the proof of [12, Theorem 1], we can show that
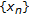 is bounded and so is
is bounded and so is  and
and  . Notice also that
. Notice also that 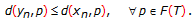 (32)
(32)
(ii) It suffices to show that

Indeed, if (3.3) holds, we obtain

By using Lemma 2.1, we get

Hence,

where  is a constant such that
is a constant such that  for all
for all  . By assumptions, we have
. By assumptions, we have

Hence, Lemma 2.5 is applicable to (3.6), and we obtain  .
.
-
(iii)
From Lemma 2.3, let
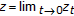 , where
, where 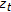 is given by (2.8). Then,
is given by (2.8). Then,  is the point of
is the point of  which is nearest
which is nearest  . We observe that
. We observe that  (38)
(38)
By Lemma 2.4, we have  for all Banach limit
for all Banach limit  . Moreover, since
. Moreover, since  ,
,

It follows from  and Lemma 2.2 that
and Lemma 2.2 that

Hence, the conclusion follows from Lemma 2.5.
By using the similar technique as in the proof of Theorem 3.1, we can obtain a strong convergence theorem which is an analog of [13, Theorem 3.1] (see also [37, 38] for subsequence comments).
Theorem 3.2.
Let C be a nonempty closed and convex subset of a complete  space
space  , and let
, and let  be a nonexpansive mapping such that
be a nonexpansive mapping such that  . Given a point
. Given a point  and an initial value
and an initial value  . The sequence
. The sequence  is defined iteratively by
is defined iteratively by

Suppose that both  and
and  are sequences in
are sequences in  satisfying
satisfying
(B1)  ,
,
(B2)  ,
,
(B3)  .
.
Then,  converges to a fixed point
converges to a fixed point  which is nearest
which is nearest  .
.
Proof.
Let  . We divide the proof into 3 steps.
. We divide the proof into 3 steps.
Step 1.
We show that  ,
,  , and
, and  are bounded sequences. Let
are bounded sequences. Let  , then we have
, then we have

Now, an induction yields

Hence,  is bounded and so are
is bounded and so are  and
and  .
.
Step 2.
We show that  . By using Lemma 2.1, we get
. By using Lemma 2.1, we get

This implies that

Since  and
and  are bounded and
are bounded and  , it follows that
, it follows that

Hence, by Lemma 2.6, we get

On the other hand,

Using (3.17) and (3.18), we get

Step 3.
We show that  converges to a fixed point of
converges to a fixed point of  . Let
. Let  , where
, where  is given by (2.8), then
is given by (2.8), then  . Finally, we show that
. Finally, we show that 

By Lemma 2.4, we have  for all Banach limit
for all Banach limit  . Moreover, since
. Moreover, since

it follows from condition  ,
,  and Lemma 2.2 that
and Lemma 2.2 that

Hence, the conclusion follows by Lemma 2.5.
References
Halpern B: Fixed points of nonexpanding maps. Bulletin of the American Mathematical Society 1967, 73: 957–961. 10.1090/S0002-9904-1967-11864-0
Reich S: Some fixed point problems. Atti della Accademia Nazionale dei Lincei 1974,57(3–4):194–198.
Lions P-L: Approximation de points fixes de contractions. Comptes Rendus de l'Académie des Sciences de Paris A-B 1977,284(21):A1357-A1359.
Reich S: Strong convergence theorems for resolvents of accretive operators in Banach spaces. Journal of Mathematical Analysis and Applications 1980,75(1):287–292. 10.1016/0022-247X(80)90323-6
Reich S: Some problems and results in fixed point theory. In Topological Methods in Nonlinear Functional Analysis (Toronto, Ont., 1982), Contemporary Mathematics. Volume 21. American Mathematical Society, Providence, RI, USA; 1983:179–187.
Takahashi W, Ueda Y: On Reich's strong convergence theorems for resolvents of accretive operators. Journal of Mathematical Analysis and Applications 1984,104(2):546–553. 10.1016/0022-247X(84)90019-2
Wittmann R: Approximation of fixed points of nonexpansive mappings. Archiv der Mathematik 1992,58(5):486–491. 10.1007/BF01190119
Shioji N, Takahashi W: Strong convergence of approximated sequences for nonexpansive mappings in Banach spaces. Proceedings of the American Mathematical Society 1997,125(12):3641–3645. 10.1090/S0002-9939-97-04033-1
Xu H-K: Iterative algorithms for nonlinear operators. Journal of the London Mathematical Society. Second Series 2002,66(1):240–256. 10.1112/S0024610702003332
Xu H-K: An iterative approach to quadratic optimization. Journal of Optimization Theory and Applications 2003,116(3):659–678. 10.1023/A:1023073621589
Xu H-K: A strong convergence theorem for contraction semigroups in Banach spaces. Bulletin of the Australian Mathematical Society 2005,72(3):371–379. 10.1017/S000497270003519X
Kim T-H, Xu H-K: Strong convergence of modified Mann iterations. Nonlinear Analysis: Theory, Methods & Applications 2005,61(1–2):51–60. 10.1016/j.na.2004.11.011
Hu L-G: Strong convergence of a modified Halpern's iteration for nonexpansive mappings. Fixed Point Theory and Applications 2008, 2008:-9.
Bridson MR, Haefliger A: Metric Spaces of Non-Positive Curvature, Grundlehren der Mathematischen Wissenschaften. Volume 319. Springer, Berlin, Germany; 1999:xxii+643.
Kirk WA: Fixed point theorems in CAT(0) spaces and -trees. Fixed Point Theory and Applications 2004,2004(4):309–316.
Brown KS: Buildings. Springer, New York, NY, USA; 1989:viii+215.
Goebel K, Reich S: Uniform Convexity, Hyperbolic Geometry, and Nonexpansive Mappings, Monographs and Textbooks in Pure and Applied Mathematics. Volume 83. Marcel Dekker Inc., New York, NY, USA; 1984:ix+170.
Kirk WA: Geodesic geometry and fixed point theory. In Seminar of Mathematical Analysis (Malaga/Seville, 2002/2003), Colección Abierta. Volume 64. University of Seville, Secretary of Publications, Seville, Spain; 2003:195–225.
Kirk WA: Geodesic geometry and fixed point theory. II. In International Conference on Fixed Point Theory and Applications. Yokohama Publishers, Yokohama, Japan; 2004:113–142.
Dhompongsa S, Kaewkhao A, Panyanak B: Lim's theorems for multivalued mappings in CAT(0) spaces. Journal of Mathematical Analysis and Applications 2005,312(2):478–487. 10.1016/j.jmaa.2005.03.055
Chaoha P, Phon-on A: A note on fixed point sets in CAT(0) spaces. Journal of Mathematical Analysis and Applications 2006,320(2):983–987. 10.1016/j.jmaa.2005.08.006
Leustean L: A quadratic rate of asymptotic regularity for CAT(0)-spaces. Journal of Mathematical Analysis and Applications 2007,325(1):386–399. 10.1016/j.jmaa.2006.01.081
Dhompongsa S, Panyanak B: On -convergence theorems in CAT(0) spaces. Computers & Mathematics with Applications 2008,56(10):2572–2579. 10.1016/j.camwa.2008.05.036
Shahzad N: Fixed point results for multimaps in CAT(0) spaces. Topology and Its Applications 2009,156(5):997–1001. 10.1016/j.topol.2008.11.016
Espinola R, Fernandez-Leon A: CAT()-spaces, weak convergence and fixed points. Journal of Mathematical Analysis and Applications 2009,353(1):410–427. 10.1016/j.jmaa.2008.12.015
Hussain N, Khamsi MA: On asymptotic pointwise contractions in metric spaces. Nonlinear Analysis: Theory, Methods & Applications 2009,71(10):4423–4429. 10.1016/j.na.2009.02.126
Laowang W, Panyanak B: Strong and convergence theorems for multivalued mappings in CAT(0) spaces. Journal of Inequalities and Applications 2009, 2009:-16.
Saejung S: Halpern's iteration in CAT(0) spaces. Fixed Point Theory and Applications 2010, 2010:-13.
Khan AR, Khamsi MA, Fukhar-Ud-Din H: Strong convergence of a general iteration scheme in CAT(0) spaces. Nonlinear Analysis: Theory, Methods and Applications 2011,74(3):783–791. 10.1016/j.na.2010.09.029
Khan SH, Abbas M: Strong and -convergence of some iterative schemes in CAT(0) spaces. Computers and Mathematics with Applications 2011,61(1):109–116. 10.1016/j.camwa.2010.10.037
Abkar A, Eslamian M: Common fixed point results in CAT(0) spaces. Nonlinear Analysis: Theory, Methods and Applications 2011,74(5):1835–1840. 10.1016/j.na.2010.10.056
Bestvina M: -trees in topology, geometry, and group theory. In Handbook of Geometric Topology. North-Holland, Amsterdam, The Netherlands; 2002:55–91.
Semple C, Steel M: Phylogenetics, Oxford Lecture Series in Mathematics and Its Applications. Volume 24. Oxford University Press, Oxford, UK; 2003:xiv+239.
Espinola R, Kirk WA: Fixed point theorems in -trees with applications to graph theory. Topology and Its Applications 2006,153(7):1046–1055. 10.1016/j.topol.2005.03.001
Kirk WA: Some recent results in metric fixed point theory. Journal of Fixed Point Theory and Applications 2007,2(2):195–207. 10.1007/s11784-007-0031-8
Suzuki T: Strong convergence theorems for infinite families of nonexpansive mappings in general Banach spaces. Fixed Point Theory and Applications 2005,2005(1):103–123.
Song Y, Chen R: Strong convergence of an iterative method for non-expansive mappings. Mathematische Nachrichten 2008,281(8):1196–1204. 10.1002/mana.200510670
Wang S: A note on strong convergence of a modified Halpern's iteration for nonexpansive mappings. Fixed Point Theory and Applications 2010, 2010:-2.
Acknowledgments
The authors are grateful to Professor Sompong Dhompongsa for his suggestions and advices during the preparation of the paper. This research was supported by the National Research University Project under Thailand's Office of the Higher Education Commission.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License (https://creativecommons.org/licenses/by/2.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
About this article
Cite this article
Cuntavepanit, A., Panyanak, B. Strong Convergence of Modified Halpern Iterations in CAT(0) Spaces. Fixed Point Theory Appl 2011, 869458 (2011). https://doi.org/10.1155/2011/869458
Received:
Accepted:
Published:
DOI: https://doi.org/10.1155/2011/869458
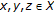 and
and  , one has
, one has 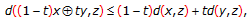
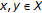 and
and 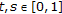 , one has
, one has 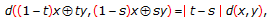
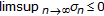 or
or  .
.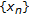 is bounded and so is
is bounded and so is  and
and  . Notice also that
. Notice also that 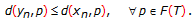
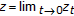 , where
, where 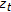 is given by (2.8). Then,
is given by (2.8). Then,  is the point of
is the point of  which is nearest
which is nearest  . We observe that
. We observe that 