- Research Article
- Open access
- Published:
Some Common Fixed Point Theorems in Menger PM Spaces
Fixed Point Theory and Applications volume 2010, Article number: 819269 (2010)
An Erratum to this article was published on 10 August 2011
Abstract
Employing the common property (E.A), we prove some common fixed point theorems for weakly compatible mappings via an implicit relation in Menger PM spaces. Some results on similar lines satisfying quasicontraction condition as well as 
-type contraction condition are also proved in Menger PM spaces. Our results substantially improve the corresponding theorems contained in (Branciari, (2002); Rhoades, (2003); Vijayaraju et al., (2005)) and also some others in Menger as well as metric spaces. Some related results are also derived besides furnishing illustrative examples.
1. Introduction and Preliminaries
Sometimes, it is found appropriate to assign the average of several measurements as a measure to ascertain the distance between two points. Inspired from this line of thinking, Menger [1, 2] introduced the notion of Probabilistic Metric spaces (in short PM spaces) as a generalization of metric spaces. In fact, he replaced the distance function 
with a distribution function 
wherein for any number 
, the value 
describes the probability that the distance between 
and 
is less than 
. In fact the study of such spaces received an impetus with the pioneering work of Schweizer and Sklar [3]. The theory of PM spaces is of paramount importance in Probabilistic Functional Analysis especially due to its extensive applications in random differential as well as random integral equations.
Fixed point theory is one of the most fruitful and effective tools in mathematics which has enormous applications within as well as outside mathematics. The theory of fixed points in PM spaces is a part of Probabilistic Analysis which continues to be an active area of mathematical research. By now, several authors have already established numerous fixed point and common fixed point theorems in PM spaces. For an idea of this kind of the literature, one can consult the results contained in [3–14].
In metric spaces, Jungck [15] introduced the notion of compatible mappings and utilized the same (as a tool) to improve commutativity conditions in common fixed point theorems. This concept has been frequently employed to prove existence theorems on common fixed points. However, the study of common fixed points of noncompatible mappings is also equally interesting which was initiated by Pant [16]. Recently, Aamri and Moutawakil [17] and Liu et al. [18] respectively, defined the property (E.A) and the common property (E.A) and proved some common fixed point theorems in metric spaces. Imdad et al. [19] extended the results of Aamri and Moutawakil [17] to semimetric spaces. Most recently, Kubiaczyk and Sharma [20] defined the property (E.A) in PM spaces and used it to prove results on common fixed points wherein authors claim to prove their results for strict contractions which are merely valid up to contractions.
In 2002, Branciari [21] proved a fixed point result for a mapping satisfying an integral-type inequality which is indeed an analogue of contraction mapping condition. In recent past, several authors (e.g., [22–26]) proved various fixed point theorems employing relatively more general integral type contractive conditions. In one of his interesting articles, Suzuki [27] pointed out that Meir-Keeler contractions of integral type are still Meir-Keeler contractions. In this paper, we prove the fixed point theorems for weakly compatible mappings via an implicit relation in Menger PM spaces satisfying the common property (E.A). Our results substantially improve the corresponding theorems contained in [21, 24, 26, 28] along with some other relevant results in Menger as well as metric spaces. Some related results are also derived besides furnishing illustrative examples.
In the following lines, we collect the background material to make our presentation as self-contained as possible.
Definition 1.1 (see [3]).
A mapping 
is called distribution function if it is nondecreasing and left continuous with 
and 
.
Let 
be the set of all distribution functions whereas 
be the set of specific distribution function (also known as Heaviside function) defined by
Definition 1.2 (see [1]).
Let 
be a nonempty set. An ordered pair 
is called a PM space if 
is a mapping from 
into 
satisfying the following conditions:
(1)
if and only if 
,
(2)
(3)
and 
, then 
, for all 
and 
Every metric space 
can always be realized as a PM space by considering 
defined by 
for all 
. So PM spaces offer a wider framework (than that of the metric spaces) and are general enough to cover even wider statistical situations.
Definition 1.3 (see [3]).
A mapping 
is called a 
-norm if
(1)
(2)
(3)
for 
(4)
for all 
.
Example 1.4.
The following are the four basic 
-norms.
(i)The minimum 
-norm: 
.
(ii)The product 
-norm: 
.
(iii)The Lukasiewicz 
-norm: 
.
(iv)The weakest 
-norm, the drastic product:
In respect of above mentioned 
-norms, we have the following ordering:
Throughout this paper, 
stands for an arbitrary continuous 
-norm.
Definition 1.5 (see [1]).
A Menger PM space 
is a triplet where 
is a PM space and 
is a 
-norm satisfying the following condition:
Definition 1.6 (see [6]).
A sequence 
in a Menger PM space 
is said to converge to a point 
in 
if for every 
and 
, there is an integer 
such that 
for all 
.
Definition 1.7 (see [10]).
A pair 
of self-mappings of a Menger PM space 
is said to be compatible if 
for all 
, whenever 
is a sequence in 
such that 
for some 
in 
as 
.
Definition 1.8 (see [23]).
A pair 
of self-mappings of a Menger PM space 
is said to be noncompatible if and only if there exists at least one sequence 
in 
such that
but for some 
is either less than 1 or nonexistent.
Definition 1.9 (see [6]).
A pair 
of self-mappings of a Menger PM space 
is said to satisfy the property (E.A) if there exist a sequence 
in 
such that
Clearly, a pair of compatible mappings as well as noncompatible mappings satisfies the property (E.A).
Inspired by Liu et al. [18], we introduce the following.
Definition 1.10.
Two pairs 
and 
of self-mappings of a Menger PM space 
are said to satisfy the common property (E.A) if there exist two sequences 
in 
and some 
in 
such that
Example 1.11.
Let 
be a Menger PM space with 
and,
for all 
. Define self-mappings 
and 
on 
as 
, 
, 
, and 
for all 
. Then with sequences 
and 
in 
, one can easily verify that
This shows that the pairs 
and 
share the common property 
Definition 1.12 (see[29]).
A pair 
of self-mappings of a nonempty set 
is said to be weakly compatible if the pair commutes on the set of coincidence points, that is, 
for some 
implies that 
.
Definition 1.13 (see [8]).
Two finite families of self-mappings 
and 
of a set 
are said to be pairwise commuting if
(1)
,
(2)
,
(3)
and 
.
2. Implicit Relation
Let 
be the set of all continuous functions 
satisfying the following conditions:
, for all 
,
, for all 
,
, for all 
.
Example 2.1.
Define 
as
where 
is increasing and continuous function such that 
for all 
Notice that
, for all 
,
, for all 
,
, for all 
.
Example 2.2.
Define 
as
where 
is an increasing and continuous function such that 
for all 
and 
is a Lebesgue integrable function which is summable and satisfies
Observe that
, for all 
,
, for all 
,
, for all 
.
Example 2.3.
Define 
as
where 
is an increasing and continuous function such that 
for all 
and 
is a Lebesgue integrable function which is summable and satisfies
Observe that
, for all 
,
, for all 
,
()
, for all 
.
3. Main Results
We begin with the following observation.
Lemma 3.1.
Let 
and 
be self-mappings of a Menger space 
satisfying the following:
(i)the pair 
(or 
satisfies the property 
;
-
(ii)
for any
and for all 
, 
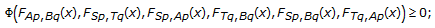(3.1)
and for all 
, 
(iii)
(or 
Then the pairs 
and 
share the common property 
Proof.
Suppose that the pair 
owns the property (E.A), then there exists a sequence 
in 
such that
Since 
, hence for each 
there exists 
such that 
Therefore,
Thus in all, we have 
and 
Now we assert that 
. Suppose that 
then applying inequality (3.1), we obtain
which on making 
reduces to
or
which is a contradiction to 
, and therefore 
. Hence the pairs 
and 
share the common property (E.A).
Remark 3.2.
The converse of Lemma 3.1 is not true in general. For a counter example, one can see Example 3.17 (presented in the end).
Theorem 3.3.
Let 
and 
be self-mappings on a Menger PM space 
satisfying inequality (3.1). Suppose that
(i)the pair 
(or 
enjoys the property (E.A),
(ii)
(or 
(iii)
(or 
is a closed subset of 
.
Then the pairs 
and 
have a point of coincidence each. Moreover, 
and 
have a unique common fixed point provided that both the pairs 
and 
are weakly compatible.
Proof.
In view of Lemma 3.1, the pairs 
and 
share the common property (E.A), that is, there exist two sequences 
and 
in 
such that
Suppose that 
is a closed subset of 
, then 
for some 
If 
, then applying inequality (3.1), we obtain
which on making 
reduces to
which is a contradiction to 
. Hence 
Since 
, there exists 
such that 
If 
, then using inequality (3.1), we have
or
which is a contradiction to 
, and therefore 
Since the pairs 
and 
are weakly compatible and 
therefore
If 
, then using inequality (3.1), we have
or
which is a contradiction to 
, and therefore 
Similarly, one can prove that 
Hence 
, and 
is a common fixed point of 
and 
. The uniqueness of common fixed point is an easy consequences of inequality (3.1).
By choosing 
and 
suitably, one can derive corollaries involving two or three mappings. As a sample, we deduce the following natural result for a pair of self-mappings by setting 
and 
(in Theorem 3.3).
Corollary 3.4.
Let 
and 
be self-mappings on a Menger space 
. Suppose that
(i)the pair 
enjoys the property (E.A),
(ii)for all 
and for all 
,
(iii)
is a closed subset of 
.
Then 
and 
have a coincidence point. Moreover, if the pair 
is weakly compatible, then 
and 
have a unique common fixed point.
Theorem 3.5.
Let 
and 
be self-mappings of a Menger PM space 
satisfying the inequality (3.1). Suppose that
(i)the pairs 
and 
share the common property (E.A),
(ii)
and 
are closed subsets of 
.
If the pairs 
and 
are weakly compatible, then 
and 
have a unique common fixed point in 
.
Proof.
Suppose that the pairs 
and 
satisfy the common property (E.A), then there exist two sequences 
and 
in 
such that
Since 
and 
are closed subsets of 
, we obtain 
for some 
.
If 
then using inequality (3.1), we have
which on making 
reduces to
which is a contradiction to 
, and hence 
The rest of the proof can be completed on the lines of the proof of Theorem 3.3, hence it is omitted.
Remark 3.6.
Theorem 3.3 extends the main result of Ciric [30] to Menger PM spaces besides extending the main result of Kubiaczyk and Sharma [20] to two pairs of mappings without any condition on containment of ranges amongst involved mappings.
Theorem 3.7.
The conclusions of Theorem 3.5 remain true if condition (ii) of Theorem 3.5 is replaced by the following:
and 
Corollary 3.8.
The conclusions of Theorems 3.3 and 3.5 remain true if conditions (ii) (of Theorem 3.3) and (iii)
(of Theorem 3.7) are replaced by the following:
(iv)
and 
are closed subsets of 
whereas 
and 
.
As an application of Theorem 3.3, we prove the following result for four finite families of self-mappings. While proving this result, we utilize Definition 1.13 which is a natural extension of commutativity condition to two finite families of mappings.
Theorem 3.9.
Let 
and 
be four finite families of self-mappings of a Menger PM space 
with 
, 
and 
satisfying condition (3.1). If the pairs 
and 
share the common property (E.A) and 
as well as 
are closed subsets of 
, then
(i)the pair 
as well as 
has a coincidence point,
(ii)
and 
have a unique common fixed point provided that the pair of families 
and 
commute pairwise, where 
, 
, 
, and 
.
Proof.
The proof follows on the lines of Theorem 4.1 according to M. Imdad and J. Ali[31] and Theorem 3.1 according to Imdad et al. [19].
Remark 3.10.
By restricting four families as 
and 
in Theorem 3.9, we can derive improved versions of certain results according to Chugh and Rathi [4], Kutukcu and Sharma [32], Rashwan and Hedar [11], Singh and Jain [14], and some others. Theorem 3.9 also generalizes the main result of Razani and Shirdaryazdi [12] to any finite number of mappings.
By setting 
and 
in Theorem 3.9, we deduce the following.
Corollary 3.11.
Let 
and 
be self-mappings of a Menger space 
such that the pairs 
and 
share the common property (E.A) and also satisfy the condition
, 
and 
and 
are fixed positive integers.
If 
and 
are closed subsets of 
, then 
and 
have a unique common fixed point provided, 
and 
.
Remark 3.12.
Corollary 3.11 is a slight but partial generalization of Theorem 3.3 as the commutativity requirements (i.e., 
and 
) in this corollary are stronger as compared to weak compatibility in Theorem 3.3. Corollary 3.11 also presents the generalized and improved form of a result according to Bryant [33] in Menger PM spaces.
Our next result involves a lower semicontinuous function 
such that 
for all 
along with 
and 
.
Theorem 3.13.
Let 
and 
be self-mappings of a Menger space 
satisfying conditions (i) and (ii) of Theorem 3.5 and for all 
where
, 
, 
, 
, 
.
Then the pairs 
and 
have point of coincidence each. Moreover, 
and 
have a unique common fixed point provided that both the pairs 
and 
are weakly compatible.
Proof.
As both the pairs share the common property 
, there exist two sequences 
such that
If 
is a closed subset of 
, then (3.21)
Therefore, there exists a point 
such that 
Now we assert that 
If it is not so, then setting 
in (3.20), we get
which on making 
, reduces to
or
a contradiction. Therefore 
, and hence 
which shows that the pair 
has a point of coincidence.
If 
is a closed subset of 
, then (3.21)
Hence, there exists a point 
such that 
Now we show that 
If it is not so, then using (3.20) with 
, we have
which on making 
reduces to
or
a contradiction. Therefore 
and hence 
which proves that the pair 
has a point of coincidence.
Since the pairs 
and 
are weakly compatible and both the pairs have point of coincidence 
and 
, respectively. Following the lines of the proof of Theorem 3.3, one can easily prove the existence of unique common fixed point of mappings 
and 
. This concludes the proof.
Remark 3.14.
Theorem 3.13 generalizes the main result of Kohli and Vashistha [9] to two pairs of self-mappings as Theorem 3.13 never requires any condition on the containment of ranges amongst involved mappings besides weakening the completeness requirement of the space to closedness of suitable subspaces along with suitable commutativity requirements of the involved mappings. Here one may also notice that the function 
is lower semicontinuous whereas all the involved mappings may be discontinuous at the same time.
Remark 3.15.
Notice that results similar to Theorems 3.5 –3.9 and Corollaries 3.4–3.11 can also be outlined in respect of Theorem 3.13, but we omit the details with a view to avoid any repetition.
We conclude this paper with two illustrative examples which demonstrate the validity of the hypotheses of Theorem 3.3 and Theorem 3.13.
Example 3.16.
Let 
be a Menger space, where 
with a 
-norm defined by 
for all 
, 
for all 
and
Define 
and 
by: 
Also define 
as
It is easy to see that for all 
and 
Also 
. Thus all the conditions of Theorem 3.3 are satisfied, and 1 is the unique common fixed point of 
and 
Example 3.17.
Let 
and 
be the same as in Example 3.16. Define 
and 
by
By a routine calculation, one can verify that for all 
and 
Also 
, 
, 
. Thus all conditions of Theorem 3.13 are satisfied, and 1 is the unique common fixed point of 
and 
References
Menger K: Statistical metrics. Proceedings of the National Academy of Sciences of the United States of America 1942, 28: 535–537. 10.1073/pnas.28.12.535
Menger K: Probabilistic geometry. Proceedings of the National Academy of Sciences of the United States of America 1951, 37: 226–229. 10.1073/pnas.37.4.226
Schweizer B, Sklar A: Probabilistic Metric Spaces, North-Holland Series in Probability and Applied Mathematics. North-Holland, New York, NY, USA; 1983:xvi+275.
Chugh R, Rathi S: Weakly compatible maps in probabilistic metric spaces. The Journal of the Indian Mathematical Society 2005,72(1–4):131–140.
Fang J, Gao Y: Common fixed point theorems under strict contractive conditions in Menger spaces. Nonlinear Analysis: Theory, Methods & Applications 2009,70(1):184–193. 10.1016/j.na.2007.11.045
Hadžić O, Pap E: Fixed Point Theory in Probabilistic Metric Spaces, Mathematics and Its Applications. Volume 536. Kluwer Academic Publishers, Dordrecht, The Netherlands; 2001:x+273.
Hicks TL: Fixed point theory in probabilistic metric spaces. Univerzitet u Novom Sadu. Zbornik Radova Prirodno-Matematičkog Fakulteta. Serija za Matemati 1983, 13: 63–72.
Imdad M, Ali J, Tanveer M: Coincidence and common fixed point theorems for nonlinear contractions in Menger PM spaces. Chaos, Solitons and Fractals 2009,42(5):3121–3129. 10.1016/j.chaos.2009.04.017
Kohli JK, Vashistha S: Common fixed point theorems in probabilistic metric spaces. Acta Mathematica Hungarica 2007,115(1–2):37–47. 10.1007/s10474-006-0533-7
Mishra SN: Common fixed points of compatible mappings in PM-spaces. Mathematica Japonica 1991,36(2):283–289.
Rashwan RA, Hedar A: On common fixed point theorems of compatible mappings in Menger spaces. Demonstratio Mathematica 1998,31(3):537–546.
Razani A, Shirdaryazdi M: A common fixed point theorem of compatible maps in Menger space. Chaos, Solitons and Fractals 2007,32(1):26–34. 10.1016/j.chaos.2005.10.096
Sehgal VM, Bharucha-Reid AT: Fixed points of contraction mappings on probabilistic metric spaces. Mathematical Systems Theory 1972, 6: 97–102. 10.1007/BF01706080
Singh B, Jain S: A fixed point theorem in Menger space through weak compatibility. Journal of Mathematical Analysis and Applications 2005,301(2):439–448. 10.1016/j.jmaa.2004.07.036
Jungck G: Compatible mappings and common fixed points. International Journal of Mathematics and Mathematical Sciences 1986,9(4):771–779. 10.1155/S0161171286000935
Pant RP: Common fixed points of noncommuting mappings. Journal of Mathematical Analysis and Applications 1994,188(2):436–440. 10.1006/jmaa.1994.1437
Aamri M, El Moutawakil D: Some new common fixed point theorems under strict contractive conditions. Journal of Mathematical Analysis and Applications 2002,270(1):181–188. 10.1016/S0022-247X(02)00059-8
Liu Y, Wu J, Li Z: Common fixed points of single-valued and multivalued maps. International Journal of Mathematics and Mathematical Sciences 2005,2005(19):3045–3055. 10.1155/IJMMS.2005.3045
Imdad M, Ali J, Khan L: Coincidence and fixed points in symmetric spaces under strict contractions. Journal of Mathematical Analysis and Applications 2006,320(1):352–360. 10.1016/j.jmaa.2005.07.004
Kubiaczyk I, Sharma S: Some common fixed point theorems in Menger space under strict contractive conditions. Southeast Asian Bulletin of Mathematics 2008,32(1):117–124.
Branciari A: A fixed point theorem for mappings satisfying a general contractive condition of integral type. International Journal of Mathematics and Mathematical Sciences 2002,29(9):531–536. 10.1155/S0161171202007524
Aliouche A: A common fixed point theorem for weakly compatible mappings in symmetric spaces satisfying a contractive condition of integral type. Journal of Mathematical Analysis and Applications 2006,322(2):796–802. 10.1016/j.jmaa.2005.09.068
Djoudi A, Aliouche A: Common fixed point theorems of Gregus type for weakly compatible mappings satisfying contractive conditions of integral type. Journal of Mathematical Analysis and Applications 2007,329(1):31–45. 10.1016/j.jmaa.2006.06.037
Rhoades BE: Two fixed-point theorems for mappings satisfying a general contractive condition of integral type. International Journal of Mathematics and Mathematical Sciences 2003, (63):4007–4013.
Turkoglu D, Altun I: A common fixed point theorem for weakly compatible mappings in symmetric spaces satisfying an implicit relation. Boletin de la Sociedad Matematica Mexicana. Tercera Serie 2007,13(1):195–205.
Vijayaraju P, Rhoades BE, Mohanraj R: A fixed point theorem for a pair of maps satisfying a general contractive condition of integral type. International Journal of Mathematics and Mathematical Sciences 2005, (15):2359–2364.
Suzuki T: Meir-Keeler contractions of integral type are still Meir-Keeler contractions. International Journal of Mathematics and Mathematical Sciences 2007, 2007:-6.
Ali J, Imdad M: An implicit function implies several contraction conditions. Sarajevo Journal of Mathematics 2008,4(17)(2):269–285.
Jungck G: Common fixed points for noncontinuous nonself maps on nonmetric spaces. Far East Journal of Mathematical Sciences 1996,4(2):199–215.
Cirić LB: A generalization of Banach's contraction principle. Proceedings of the American Mathematical Society 1974, 45: 267–273.
Imdad M, Ali J: Jungck's common fixed point theorem and E.A property. Acta Mathematica Sinica 2008,24(1):87–94. 10.1007/s10114-007-0990-0
Kutukcu S, Sharma S: Compatible maps and common fixed points in Menger probabilistic metric spaces. Communications of the Korean Mathematical Society 2009,24(1):17–27. 10.4134/CKMS.2009.24.1.017
Bryant VW: A remark on a fixed-point theorem for iterated mappings. The American Mathematical Monthly 1968, 75: 399–400.
Author information
Authors and Affiliations
Corresponding author
Additional information
An erratum to this article is available at http://dx.doi.org/10.1186/1687-1812-2011-28.
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License ( https://creativecommons.org/licenses/by/2.0 ), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
About this article
Cite this article
Imdad, M., Tanveer, M. & Hasan, M. Some Common Fixed Point Theorems in Menger PM Spaces. Fixed Point Theory Appl 2010, 819269 (2010). https://doi.org/10.1155/2010/819269
Received:
Accepted:
Published:
DOI: https://doi.org/10.1155/2010/819269