- Research Article
- Open access
- Published:
On Some Geometric Constants and the Fixed Point Property for Multivalued Nonexpansive Mappings
Fixed Point Theory and Applications volume 2010, Article number: 596952 (2010)
Abstract
We show some geometric conditions on a Banach space X concerning the Jordan-von Neumann constant, Zbăganu constant, characteristic of (separation) noncompact convexity, and the coefficient R(1, X), the weakly convergent sequence coefficient, which imply the existence of fixed points for multivalued nonexpansive mappings.
1. Introduction
Fixed point theory for multivalued mappings has many useful applications in Applied Sciences, in particular, in game theory and mathematical economics. Thus it is natural to try of extending the known fixed point results for single-valued mappings to the setting of multivalued mappings.
In 1969, Nadler [1] established the multivalued version of Banach's contraction principle. One of the most celebrated results about multivalued mappings was given by Lim [2] in 1974. Using Edelstein's method of asymptotic centers, he proved the existence of a fixed point for a multivalued nonexpansive self-mapping  where
where  is a nonempty bounded closed convex subset of a uniformly convex Banach space. Since then the metric fixed point theory of multivalued mappings has been rapidly developed. Some other classical fixed point theorems for single-valued mappings have been extended to multivalued mappings. However, many questions remain open, for instance, the possibility of extending the well-known Kirk's theorem, that is, do Banach spaces with weak normal structure have the fixed point property (FPP, in short) for multivalued nonexpansive mappings?
is a nonempty bounded closed convex subset of a uniformly convex Banach space. Since then the metric fixed point theory of multivalued mappings has been rapidly developed. Some other classical fixed point theorems for single-valued mappings have been extended to multivalued mappings. However, many questions remain open, for instance, the possibility of extending the well-known Kirk's theorem, that is, do Banach spaces with weak normal structure have the fixed point property (FPP, in short) for multivalued nonexpansive mappings?
Since weak normal structure is implied by different geometrical properties of Banach spaces, it is natural to study if those properties imply the FPP for multivalued mappings. Dhompongsa et al. [3, 4] introduced the Domnguez-Lorenzo condition ((DL) condition, in short) and property (D) which imply the FPP for multivalued nonexpansive mappings. A possible approach to the above problem is to look for geometric conditions in a Banach space X which imply either the (DL) condition or property (D). In this setting the following results have been obtained.
-
(1)
Dhompongsa et al. [3] proved that uniformly nonsquare Banach spaces with property WORTH satisfy the (DL) condition.
-
(2)
Dhompongsa et al. [4] showed that the condition
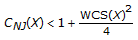 (1.1)
(1.1)
implies property (D).
(3)Satit Saejung [5] proved that the condition  implies property (D).
implies property (D).
(4)Gavira [6] showed that the condition

implies (DL) condition.
In 2007, Domínguez Benavides and Gavira [7] have established FFP for multivalued nonexpansive mappings in terms of the modulus of squareness, universal infinite-dimensional modulus, and Opia modulus. Attapol Kaewkhao [8] has established FFP for multivalued nonexpansive mappings in terms of the James constant, the Jordan-von Neumann Constants, weak orthogonality.
Besides, In 2010, Domínguez Benavides and Gavira [9] have given a survey of this subject and presented the main known results and current research directions.
In this paper, in terms of the Jordan-von Neumann constant, Zb ganu constant,
ganu constant,  and the coefficient
and the coefficient  , the weakly convergent sequence coefficient, we show some geometrical properties which imply the property (D) or (DL) condition and so the FPP for multivalued nonexpansive mappings.
, the weakly convergent sequence coefficient, we show some geometrical properties which imply the property (D) or (DL) condition and so the FPP for multivalued nonexpansive mappings.
2. Preliminaries
Let  be a Banach space and
be a Banach space and  be a nonempty subset of
be a nonempty subset of  ; we denote all nonempty bounded closed subsets of
; we denote all nonempty bounded closed subsets of  by
by  and all nonempty compact convex subsets of
and all nonempty compact convex subsets of  by
by  .
.
A multivalued mapping  is said to be nonexpansive if the inequality
is said to be nonexpansive if the inequality

holds for every  , where
, where  is the Hausdorff distance on
is the Hausdorff distance on  , that is,
, that is,

Let  be a nonempty bounded closed convex subset and
be a nonempty bounded closed convex subset and  a bounded sequence; we use
a bounded sequence; we use  and
and  to denote the asymptotic radius and the asymptotic center of
to denote the asymptotic radius and the asymptotic center of  in
in  , respectively, that is,
, respectively, that is,

It is known that  is a nonempty weakly compact convex as
is a nonempty weakly compact convex as  is.
is.
Let  and
and  be as above; then
be as above; then  is called regular relative to
is called regular relative to  if
if  for all subsequence
for all subsequence  of
of  ; further,
; further,  is called asymptotically uniform relative to
is called asymptotically uniform relative to  if
if  for all subsequence
for all subsequence  of
of  . In Banach spaces, we have the following results:
. In Banach spaces, we have the following results:
(1)(Goebel [10] and Lim [2]) there always exists a subsequence of  which is regular relative to
which is regular relative to  ;
;
-
(2)
(Kirk [11]) if
 is separable, then
is separable, then 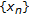 contains a subsequence which is asymptotically uniform relative to
contains a subsequence which is asymptotically uniform relative to  .
.
If  is a bounded subset of
is a bounded subset of  , the Chebyshev radius of
, the Chebyshev radius of  relative to
relative to  is defined by
is defined by

In 2006, Dhompongsa et al. [3] introduced the Domnguez-Lorenzo condition ((DL) condition, in short) in the following way.
Definition 2.1 (see [3]).
We say that a Banach space  satisfies the (DL) condition if there exists
satisfies the (DL) condition if there exists  such that for every weakly compact convex subset
such that for every weakly compact convex subset  of
of  and for every bounded sequence
and for every bounded sequence  in
in  which is regular with respect to
which is regular with respect to  ,
,

The (DL) condition implies weak normal structure [3]. We recll that a Banach space  is said to have a weak normal structure (w-NS) if for every weakly compact convex subset
is said to have a weak normal structure (w-NS) if for every weakly compact convex subset  of
of  with
with  there exist
there exist  such that
such that  .
.
The (DL) condition also implies the existence of fixed points for multivalued nonexpansive mappings.
Theorem 2.2 (see [3]).
Let  be a weakly compact convex subset of Banach space
be a weakly compact convex subset of Banach space  ; if
; if  satisfies (DL) condition, then multivalued nonexpansive mapping
satisfies (DL) condition, then multivalued nonexpansive mapping  has a fixed point.
has a fixed point.
Definition 2.3 (see [4]).
A Banach space  is said to have property (D) if there exists
is said to have property (D) if there exists  such that for every weakly compact convex subset
such that for every weakly compact convex subset  of
of  and for every sequence
and for every sequence  and for every
and for every  which are regular asymptotically uniform relative to
which are regular asymptotically uniform relative to  ,
,

It was observed that property (D) is weaker than the (DL) condition and stronger than weak normal structure, and Dhompongsa et al. [4] proved that property (D) implies the w-MFPP.
Theorem 2.4 (see [4]).
Let  be a weakly compact convex subset of Banach space
be a weakly compact convex subset of Banach space  ; if
; if  satisfies property (D), then multivalued nonexpansive mapping
satisfies property (D), then multivalued nonexpansive mapping  has a fixed point.
has a fixed point.
Before going to the results, let us recall some more definitions. Let  be a Banach space.
be a Banach space.
The Benavides coefficient  is defined by Domínguez Benavides [12] as
is defined by Domínguez Benavides [12] as

where the supremum is taken over all  with
with  and all weakly null sequence
and all weakly null sequence  in
in  such that
such that

Obviously,  .
.
The weakly convergent sequence coefficient  is equivalently defined by (see [13])
is equivalently defined by (see [13])

where the infimum is taken over all weakly (not strongly) null sequences  with
with  existing.
existing.
The ultrapower of a Banach space has proved to be useful in many branches of mathematics. Many results can be seen more easily when treated in this setting.
First we recall some basic facts about ultrapowers. Let  be a filter on an index set
be a filter on an index set  and let
and let  be a Banach space. A sequence
be a Banach space. A sequence  in
in  convergers to
convergers to with respect to
with respect to , denoted by
, denoted by  , if for each neighborhood
, if for each neighborhood  of
of  ,
,  . A filter
. A filter  on
on  is called an ultrafilter if it is maximal with respect to the set inclusion. An ultrafilter is called trivial if it is of the form
is called an ultrafilter if it is maximal with respect to the set inclusion. An ultrafilter is called trivial if it is of the form  for some fixed
for some fixed  ; otherwise, it is called nontrivial. Let
; otherwise, it is called nontrivial. Let  denote the subspace of the product space
denote the subspace of the product space  equipped with the norm
equipped with the norm

Let  be an ultrafilter on
be an ultrafilter on  and let
and let

The ultrapower of  , denoted by
, denoted by  , is the quotient space
, is the quotient space  equipped with the quotient norm. Write
equipped with the quotient norm. Write  to denote the elements of ultrapower. It follows from the definition of the quotient norm that
to denote the elements of ultrapower. It follows from the definition of the quotient norm that

Note that if  is nontrivial, then
is nontrivial, then  can be embedded into
can be embedded into  isometrically. For more details see [14].
isometrically. For more details see [14].
3. Main Results
We first give some sufficient conditions which imply (DL) condition. The Jordan-von Neumann constant  was defined in 1937 by Clarkson [15] as
was defined in 1937 by Clarkson [15] as

Theorem 3.1.
Let  be a Banach space and
be a Banach space and  a weakly compact convex subset of
a weakly compact convex subset of  . Assume that
. Assume that  is a bounded sequence in
is a bounded sequence in  which is regulary relative to
which is regulary relative to  . Then
. Then

Proof.
Denote  and
and  . We can assume that
. We can assume that  . Since
. Since  is bounded and
is bounded and  is a weakly compact set, by passing through a subsequence if necessary, we can also assume that
is a weakly compact set, by passing through a subsequence if necessary, we can also assume that  converges weakly to some element in
converges weakly to some element in  and
and  exists. We note that since
exists. We note that since  is regular,
is regular,  for any subsequence
for any subsequence  of
of  . Observe that, since the norm is weak lower semicontinuity, we have
. Observe that, since the norm is weak lower semicontinuity, we have

Let  ; taking a subsequence if necessary, we can assume that
; taking a subsequence if necessary, we can assume that  for all
for all  .
.
Let  . Then we have
. Then we have  and
and  . Denote
. Denote  ; by definition, we have
; by definition, we have

On the other hand, observe that the convexity of  implies
implies  ; since the norm is weak lower semicontinuity, we have
; since the norm is weak lower semicontinuity, we have

In the ultrapower  of
of  , we consider
, we consider

Using the above estimates, we obtain

Therefore, we have

Since Jordan-von Neumann constant  of
of  equals to
equals to  of
of  , we obtain
, we obtain

Hence we deduce the desired inequality.
By Theorems 2.2 and 3.1, we have the following result.
Corollary 3.2.
Let  be a nonempty bounded closed convex subset of a Banach space
be a nonempty bounded closed convex subset of a Banach space  such that
such that  and
and  a nonexpansive mapping. Then
a nonexpansive mapping. Then  has a fixed point.
has a fixed point.
Proof.
since  , if
, if  , then we have
, then we have  which implies that
which implies that  is uniformly nonsquare; hence
is uniformly nonsquare; hence  is reflexive. Thus by Theorems 2.2 and 3.1, the result follows.
is reflexive. Thus by Theorems 2.2 and 3.1, the result follows.
Remark 3.3.
Note that  ; it is easy to see that Theorem 3.1 includes [6, Theorem
; it is easy to see that Theorem 3.1 includes [6, Theorem  ] and Corollary 3.2 includes [6, Corollary
] and Corollary 3.2 includes [6, Corollary  ].
].
To characterize Hilbert space, Zb ganu defined the following Zb
ganu defined the following Zb ganu constant: (see [16])
ganu constant: (see [16])

We first give the following tool.
Proposition 3.4.
 .
.
Proof.
Clearly,  . To show
. To show  , suppose
, suppose  are not all zero. Without loss of generality, we assume
are not all zero. Without loss of generality, we assume  .
.
Let us choose  . Since
. Since  and
and

the set  belongs to
belongs to  . In particular, noticing that
. In particular, noticing that  for
for  , there exists
, there exists  such that
such that

Hence, the inequality  follows from the arbitrariness of
follows from the arbitrariness of  .
.
Theorem 3.5.
Let  be a Banach space and
be a Banach space and  a weakly compact convex subset of
a weakly compact convex subset of  . Assume that
. Assume that  is a bounded sequence in
is a bounded sequence in  which is regulary relative to
which is regulary relative to  . Then
. Then

Proof.
Let  be as in Theorem 3.1. Then
be as in Theorem 3.1. Then

Therefore, by the definition of Zb ganu constant, we have
ganu constant, we have

Since Zb ganu constant
ganu constant  of
of  equals to
equals to  of
of  , we obtain
, we obtain

Hence we deduce the desired inequality.
Using Theorem 2.2, we obtain the following corollary.
Corollary 3.6.
Let  be a nonempty weakly compact convex subset of a Banach space
be a nonempty weakly compact convex subset of a Banach space  such that
such that  and let
and let  be a nonexpansive mapping. Then
be a nonexpansive mapping. Then  has a fixed point.
has a fixed point.
In the following, we present some properties concerning geometrical constants of Banach spaces which also imply the property (D).
Theorem 3.7.
Let  be a Banach space. If
be a Banach space. If  ; then
; then  has property (D).
has property (D).
Proof.
Let  be a weakly compact convex subset of
be a weakly compact convex subset of  ; suppose that
; suppose that  and
and  are regular and asymptotically uniform relative to
are regular and asymptotically uniform relative to  . Passing to a subsequence of
. Passing to a subsequence of  , still denoted by
, still denoted by  , we may assume that
, we may assume that  and
and  exists.
exists.
Let  . Again passing to a subsequence of
. Again passing to a subsequence of  , still denoted by
, still denoted by  , we assume in addition that
, we assume in addition that

Let us consider an ultrapower  of
of  . Put
. Put

then we know that  . We see that
. We see that


Thus, By the definition of Zb ganu constant, we have
ganu constant, we have

Since the Zb ganu constants of
ganu constants of  and of
and of  are the same, we obtain
are the same, we obtain  . Now we estimate
. Now we estimate  as follows:
as follows:

Hence  and the assertion follows by the definition of property (D).
and the assertion follows by the definition of property (D).
Using Theorems 2.4 and 3.7, we obtain the follwing corollary.
Corollary 3.8.
Let  be a nonempty bounded closed convex subset of a reflexive Banach space
be a nonempty bounded closed convex subset of a reflexive Banach space  such that
such that  and let
and let  be a nonexpansive mapping. Then
be a nonexpansive mapping. Then  has a fixed point.
has a fixed point.
The separation measure of noncompactness is defined by

for any bounded subset  of a Banach space
of a Banach space  , where
, where

The modulus of noncompact convexity associated to  is defined in the following way:
is defined in the following way:

The characteristic of noncompact convexity of  associated with the measure of noncompactness
associated with the measure of noncompactness  is defined by
is defined by

When  is a reflexive Banach space, we have the following alternative expression for the modulus of noncompact convexity associated with
is a reflexive Banach space, we have the following alternative expression for the modulus of noncompact convexity associated with  ,
,

It is known that  is NUC if and only if
is NUC if and only if  . The above-mentioned definitions and properties can be found in [17].
. The above-mentioned definitions and properties can be found in [17].
Theorem 3.9.
Let  be a reflexive Banach space. If
be a reflexive Banach space. If  , then
, then  has property (D).
has property (D).
Proof.
Let  be a weakly compact convex subset of
be a weakly compact convex subset of  ; suppose that
; suppose that  and
and  are regular and asymptotically uniform relative to
are regular and asymptotically uniform relative to  . Passing to a subsequence of
. Passing to a subsequence of  , still denoted by
, still denoted by  , we may assume that
, we may assume that  and
and  exists. Let
exists. Let  .
.
Since  , we have
, we have

So for any  , there exists
, there exists  such that
such that  and
and  for all
for all  .
.
Without loss of generality, we suppose that  for all
for all  . Now we consider sequence
. Now we consider sequence  ; notice that
; notice that

By the definition of  , we have
, we have

Since the last inequality is true for any  , we obtain
, we obtain  ; thus
; thus  . Now we estimate
. Now we estimate  as follows:
as follows:

Hence,

Remark 3.10.
Since  , Theorem 3.9 implies the [5, Theorem
, Theorem 3.9 implies the [5, Theorem  ]. Furthermore, it is easy to see
]. Furthermore, it is easy to see  ; then Theorem 3.9 also includes [4, Theorem
; then Theorem 3.9 also includes [4, Theorem  ].
].
By Theorem 3.9, we obtain the following Corollary.
Corollary 3.11.
Let  be a nonempty bounded closed convex subset of a reflexive Banach space
be a nonempty bounded closed convex subset of a reflexive Banach space  such that
such that  and let
and let  be a nonexpansive mapping. Then
be a nonexpansive mapping. Then  has a fixed point.
has a fixed point.
Noticing  , obviously, Corollary 3.11 extends the following well-known result.
, obviously, Corollary 3.11 extends the following well-known result.
Theorem 3.12 (see [18, Theorem  ]).
]).
Let  be a nonempty bounded closed convex subset of a reflexive Banach space
be a nonempty bounded closed convex subset of a reflexive Banach space  such that
such that  and let
and let  be a nonexpansive mapping. Then
be a nonexpansive mapping. Then  has a fixed point.
has a fixed point.
References
Nadler SB Jr.: Multi-valued contraction mappings. Pacific Journal of Mathematics 1969, 30: 475–488.
Lim TC: A fixed point theorem for multivalued nonexpansive mappings in a uniformly convex Banach space. Bulletin of the American Mathematical Society 1974, 80: 1123–1126. 10.1090/S0002-9904-1974-13640-2
Dhompongsa S, Kaewcharoen A, Kaewkhao A: The Domínguez-Lorenzo condition and multivalued nonexpansive mappings. Nonlinear Analysis: Theory, Methods & Applications 2006,64(5):958–970. 10.1016/j.na.2005.05.051
Dhompongsa S, Domínguez Benavides T, Kaewcharoen A, Kaewkhao A, Panyanak B: The Jordan-von Neumann constants and fixed points for multivalued nonexpansive mappings. Journal of Mathematical Analysis and Applications 2006,320(2):916–927. 10.1016/j.jmaa.2005.07.063
Saejung S: Remarks on sufficient conditions for fixed points of multivalued nonexpansive mappings. Nonlinear Analysis: Theory, Methods & Applications 2007,67(5):1649–1653. 10.1016/j.na.2006.07.037
Gavira B: Some geometric conditions which imply the fixed point property for multivalued nonexpansive mappings. Journal of Mathematical Analysis and Applications 2008,339(1):680–690. 10.1016/j.jmaa.2007.07.015
Domínguez Benavides T, Gavira B: The fixed point property for multivalued nonexpansive mappings. Journal of Mathematical Analysis and Applications 2007,328(2):1471–1483. 10.1016/j.jmaa.2006.06.059
Kaewkhao A: The James constant, the Jordan-von Neumann constant, weak orthogonality, and fixed points for multivalued mappings. Journal of Mathematical Analysis and Applications 2007,333(2):950–958. 10.1016/j.jmaa.2006.12.001
Domínguez Benavides T, Gavira B: Does Kirk's theorem hold for multivalued nonexpansive mappings? Fixed Point Theory and Applications 2010, 2010:-20.
Goebel K: On a fixed point theorem for multivalued nonexpansive mappings. Annales Universitatis Mariae Curie-Sklodowska 1975, 29: 69–72.
Kirk WA: Nonexpansive mappings in product spaces, set-valued mappings and k -uniform rotundity. In Nonlinear Functional Analysis and Its Applications, Proc. Sympos. Pure Math.. Volume 45. Amer. Math. Soc., Providence, RI, USA; 1986:51–64.
Dominguez Benavides T: A geometrical coefficient implying the fixed point property and stability results. Houston Journal of Mathematics 1996,22(4):835–849.
Sims B, Smyth MA: On some Banach space properties sufficient for weak normal structure and their permanence properties. Transactions of the American Mathematical Society 1999,351(2):497–513. 10.1090/S0002-9947-99-01862-0
Sims B: "Ultra"-Techniques in Banach Space Theory, Queen's Papers in Pure and Applied Mathematics. Volume 60. Queen's University, Kingston, Canada; 1982:iv+117.
Clarkson JA: The von Neumann-Jordan constant for the Lebesgue spaces. Annals of Mathematics 1937,38(1):114–115. 10.2307/1968512
Zbăganu G: An inequality of M. Rădulescu and S. Rădulescu which characterizes the inner product spaces. Revue Roumaine de Mathématiques Pures et Appliquées 2002,47(2):253–257.
Ayerbe Toledano JM, Domínguez Benavides T, López Acedo G: Measures of Noncompactness in Metric Fixed Point Theory, Operator Theory: Advances and Applications. Volume 99. Birkhäuser, Basel, Switzerland; 1997:viii+211.
Domínguez Benavides T, Lorenzo Ramírez P: Asymptotic centers and fixed points for multivalued nonexpansive mappings. Annales Universitatis Mariae Curie-Sk lodowska. Sectio A 2004, 58: 37–45.
Acknowledgments
The authors would like to thank the anonymous referee for providing some suggestions to improve the manuscript. This work was supported by China Natural Science Fund under grant 10571037.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License (https://creativecommons.org/licenses/by/2.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
About this article
Cite this article
Zhang, J., Cui, Y. On Some Geometric Constants and the Fixed Point Property for Multivalued Nonexpansive Mappings. Fixed Point Theory Appl 2010, 596952 (2010). https://doi.org/10.1155/2010/596952
Received:
Accepted:
Published:
DOI: https://doi.org/10.1155/2010/596952
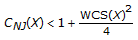
 is separable, then
is separable, then 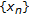 contains a subsequence which is asymptotically uniform relative to
contains a subsequence which is asymptotically uniform relative to  .
.