- Research Article
- Open access
- Published:
Generalized Asymptotic Pointwise Contractions and Nonexpansive Mappings Involving Orbits
Fixed Point Theory and Applications volume 2010, Article number: 458265 (2009)
Abstract
We give fixed point results for classes of mappings that generalize pointwise contractions, asymptotic contractions, asymptotic pointwise contractions, and nonexpansive and asymptotic nonexpansive mappings. We consider the case of metric spaces and, in particular, CAT spaces. We also study the well-posedness of these fixed point problems.
spaces. We also study the well-posedness of these fixed point problems.
1. Introduction
Four recent papers [1–4] present simple and elegant proofs of fixed point results for pointwise contractions, asymptotic pointwise contractions, and asymptotic nonexpansive mappings. Kirk and Xu [1] study these mappings in the context of weakly compact convex subsets of Banach spaces, respectively, in uniformly convex Banach spaces. Hussain and Khamsi [2] consider these problems in the framework of metric spaces and  spaces. In [3], the authors prove coincidence results for asymptotic pointwise nonexpansive mappings. Espínola et al. [4] examine the existence of fixed points and convergence of iterates for asymptotic pointwise contractions in uniformly convex metric spaces.
spaces. In [3], the authors prove coincidence results for asymptotic pointwise nonexpansive mappings. Espínola et al. [4] examine the existence of fixed points and convergence of iterates for asymptotic pointwise contractions in uniformly convex metric spaces.
In this paper we do not consider more general spaces, but instead we formulate less restrictive conditions for the mappings and show that the conclusions of the theorems still stand even in such weaker settings.
2. Preliminaries
Let  be a metric space. For
be a metric space. For  and
and  we denote the closed ball centered at
we denote the closed ball centered at  with radius
with radius  by
by  .
.
Let  and let
and let  . Throughout this paper we will denote the fixed point set of
. Throughout this paper we will denote the fixed point set of  by
by  . The mapping
. The mapping  is called a Picard operator if it has a unique fixed point
is called a Picard operator if it has a unique fixed point  and
and  converges to
converges to  for each
for each  .
.
A sequence  is said to be an approximate fixed point sequence for the mapping
is said to be an approximate fixed point sequence for the mapping  if
if 
The fixed point problem for  is well-posed (see [5, 6]) if
is well-posed (see [5, 6]) if  has a unique fixed point and every approximate fixed point sequence converges to the unique fixed point of
has a unique fixed point and every approximate fixed point sequence converges to the unique fixed point of  .
.
A mapping  is called a pointwise contraction if there exists a function
is called a pointwise contraction if there exists a function  such that
such that

Let  and for
and for  let
let  such that
such that

If the sequence  converges pointwise to the function
converges pointwise to the function  , then
, then  is called an asymptotic pointwise contraction.
is called an asymptotic pointwise contraction.
If for every  ,
,  , then
, then  is called an asymptotic pointwise nonexpansive mapping.
is called an asymptotic pointwise nonexpansive mapping.
If there exists  such that for every
such that for every  ,
,  , then
, then  is called a strongly asymptotic pointwise contraction.
is called a strongly asymptotic pointwise contraction.
For a mapping  and
and  we define the orbit starting at
we define the orbit starting at  by
by

where  for
for  and
and  . Denote also
. Denote also 
Given  and
and  , the number
, the number  is called the radius of
is called the radius of  relative to
relative to  . The diameter of
. The diameter of  is
is  and the cover of
and the cover of  is defined as
is defined as 
As in [2], we say that a family  of subsets of
of subsets of  defines a convexity structure on
defines a convexity structure on  if it contains the closed balls and is stable by intersection. A subset of
if it contains the closed balls and is stable by intersection. A subset of  is admissible if it is a nonempty intersection of closed balls. The class of admissible subsets of
is admissible if it is a nonempty intersection of closed balls. The class of admissible subsets of  denoted by
denoted by  defines a convexity structure on
defines a convexity structure on  . A convexity structure
. A convexity structure  is called compact if any family
is called compact if any family  of elements of
of elements of  has nonempty intersection provided
has nonempty intersection provided  for any finite subset
for any finite subset  .
.
According to [2], for a convexity structure  , a function
, a function  is called
is called  -convex if
-convex if  for any
for any  . A type is defined as
. A type is defined as  where
where  is a bounded sequence in
is a bounded sequence in  . A convexity structure
. A convexity structure  is
is  -stable if all types are
-stable if all types are  -convex.
-convex.
The following lemma is mentioned in [2].
Lemma 2.1.
Let  be a metric space and
be a metric space and  a compact convexity structure on
a compact convexity structure on  which is
which is  -stable. Then for any type
-stable. Then for any type  there is
there is  such that
such that

A metric space  is a geodesic space if every two points
is a geodesic space if every two points  can be joined by a geodesic. A geodesic from
can be joined by a geodesic. A geodesic from  to
to  is a mapping
is a mapping  , where
, where  , such that
, such that  and
and  for every
for every  . The image
. The image  of
of  forms a geodesic segment which joins
forms a geodesic segment which joins  and
and  . A geodesic triangle
. A geodesic triangle  consists of three points
consists of three points  and
and  in
in  (the vertices of the triangle) and three geodesic segments corresponding to each pair of points (the edges of the triangle). For the geodesic traingle
(the vertices of the triangle) and three geodesic segments corresponding to each pair of points (the edges of the triangle). For the geodesic traingle  , a comparison triangle is the triangle
, a comparison triangle is the triangle  in the Euclidean space
in the Euclidean space  such that
such that  for
for  . A geodesic triangle
. A geodesic triangle  satisfies the
satisfies the  inequality if for every comparison triangle
inequality if for every comparison triangle  of
of  and for every
and for every  we have
we have

where  are the comparison points of
are the comparison points of  and
and  . A geodesic metric space is a
. A geodesic metric space is a  space if every geodesic traingle satisfies the
space if every geodesic traingle satisfies the  inequality. In a similar way we can define
inequality. In a similar way we can define  spaces for
spaces for  or
or  using the model spaces
using the model spaces  .
.
A geodesic space is a  space if and only if it satisfies the following inequality known as the (CN) inequality of Bruhat and Tits [7]. Let
space if and only if it satisfies the following inequality known as the (CN) inequality of Bruhat and Tits [7]. Let  be points of a
be points of a  space and let
space and let  be the midpoint of
be the midpoint of  . Then
. Then

It is also known (see [8]) that in a complete  space, respectively, in a closed convex subset of a complete
space, respectively, in a closed convex subset of a complete  space every type attains its infimum at a single point. For more details about
space every type attains its infimum at a single point. For more details about  spaces one can consult, for instance, the papers [9, 10].
spaces one can consult, for instance, the papers [9, 10].
In [2], the authors prove the following fixed point theorems.
Theorem 2.2.
Let  be a bounded metric space. Assume that the convexity structure
be a bounded metric space. Assume that the convexity structure  is compact. Let
is compact. Let  be a pointwise contraction. Then
be a pointwise contraction. Then  is a Picard operator.
is a Picard operator.
Theorem 2.3.
Let  be a bounded metric space. Assume that the convexity structure
be a bounded metric space. Assume that the convexity structure  is compact. Let
is compact. Let  be a strongly asymptotic pointwise contraction. Then
be a strongly asymptotic pointwise contraction. Then  is a Picard operator.
is a Picard operator.
Theorem 2.4.
Let  be a bounded metric space. Assume that there exists a convexity structure
be a bounded metric space. Assume that there exists a convexity structure  that is compact and
that is compact and  -stable. Let
-stable. Let  be an asymptotic pointwise contraction. Then
be an asymptotic pointwise contraction. Then  is a Picard operator.
is a Picard operator.
Theorem 2.5.
Let  be a complete
be a complete  space and let
space and let  be a nonempty, bounded, closed and convex subset of
be a nonempty, bounded, closed and convex subset of  . Then any mapping
. Then any mapping  that is asymptotic pointwise nonexpansive has a fixed point. Moreover,
that is asymptotic pointwise nonexpansive has a fixed point. Moreover,  is closed and convex.
is closed and convex.
The purpose of this paper is to present fixed point theorems for mappings that satisfy more general conditions than the ones which appear in the classical definitions of pointwise contractions, asymptotic contractions, asymptotic pointwise contractions and asymptotic nonexpansive mappings. Besides this, we show that the fixed point problems are well-posed. Some generalizations of nonexpansive mappings are also considered. We work in the context of metric spaces and  spaces.
spaces.
3. Generalizations Using the Radius of the Orbit
In the sequel we extend the results obtained by Hussain and Khamsi [2] using the radius of the orbit. We also study the well-posedness of the fixed point problem. We start by introducing a property for a mapping  , where
, where  is a metric space. Namely, we will say that
is a metric space. Namely, we will say that  satisfies property
satisfies property  if
if
(S)for every approximate fixed point sequence  and for every
and for every  the sequence
the sequence  converges to 0 uniformly with respect to m.
converges to 0 uniformly with respect to m.
For instance, if for every  ,
, then property
then property  is fulfilled.
is fulfilled.
Proposition 3.1.
Let  be a metric space and let
be a metric space and let  be a mapping which satisfies
be a mapping which satisfies  . If
. If  is an approximate fixed point sequence, then for every
is an approximate fixed point sequence, then for every  and every
and every  ,
,



Proof.
Since  satisfies
satisfies  and
and  is an approximate fixed point sequence, it easily follows that (3.1) holds. To prove (3.2), let
is an approximate fixed point sequence, it easily follows that (3.1) holds. To prove (3.2), let  . Then there exists
. Then there exists  such that
such that

Taking the superior limit,

Hence, (3.2) holds. Now let again  . Then there exist
. Then there exist  such that
such that

We only need to let  in the above relation to prove (3.3).
in the above relation to prove (3.3).
Theorem 3.2.
Let  be a bounded metric space such that
be a bounded metric space such that  is compact. Also let
is compact. Also let  for which there exists
for which there exists  such that
such that

Then  is a Picard operator. Moreover, if additionally
is a Picard operator. Moreover, if additionally  satisfies
satisfies  , then the fixed point problem is well-posed.
, then the fixed point problem is well-posed.
Proof.
Because  is compact, there exists a nonempty minimal
is compact, there exists a nonempty minimal  -invariant
-invariant  for which
for which  . If
. If  then
then  In a similar way as in the proof of Theorem 3.1 of [2] we show now that
In a similar way as in the proof of Theorem 3.1 of [2] we show now that  has a fixed point. Let
has a fixed point. Let  . Then,
. Then,

This means that  , so
, so  Therefore,
Therefore,

Denote

 since it is nonempty and
since it is nonempty and 
Let  . As above we have
. As above we have  and hence
and hence  Because
Because  is minimal
is minimal  -invariant it follows that
-invariant it follows that  . This yields
. This yields  for every
for every  . In particular,
. In particular,  and using (3.9) we obtain
and using (3.9) we obtain  which implies that
which implies that  consists of exactly one point which will be fixed under
consists of exactly one point which will be fixed under  .
.
Now suppose  are fixed points of
are fixed points of  . Then
. Then

This means that  which is impossible.
which is impossible.
Let  denote the unique fixed point of
denote the unique fixed point of  , let
, let  and
and  Observe that the sequence
Observe that the sequence  is decreasing and bounded below by
is decreasing and bounded below by  so its limit exists and is precisely
so its limit exists and is precisely  . Then
. Then

This implies that  and hence
and hence 
Next we prove that the problem is well-posed. Let  be an approximate fixed point sequence. We know that
be an approximate fixed point sequence. We know that

Taking the superior limit and applying (3.2) of Proposition 3.1 for  ,
,

which implies  .
.
We remark that if in the above result  is, in particular, a pointwise contraction then the fixed point problem is well-posed without additional assumptions for
is, in particular, a pointwise contraction then the fixed point problem is well-posed without additional assumptions for  .
.
Next we give an example of a mapping which is not a pointwise contraction, but fulfills (3.7).
Example 3.3.
Let  ,
,

and let 

Then  is not a pointwise contraction, but (3.7) is verified.
is not a pointwise contraction, but (3.7) is verified.
Proof.
 is not continuous, so it is not nonexpansive and hence it cannot be a pointwise contraction. If
is not continuous, so it is not nonexpansive and hence it cannot be a pointwise contraction. If  or
or  the conclusion is immediate. Suppose
the conclusion is immediate. Suppose  and
and  . Then
. Then

(i)If  , then
, then

The above is true because 
(ii)If  , then
, then

Theorem 3.4.
Let  be a bounded metric space,
be a bounded metric space,  and suppose there exists a convexity structure
and suppose there exists a convexity structure  which is compact and
which is compact and  -stable. Assume
-stable. Assume

where for each  and the sequence
and the sequence  converges pointwise to a function
converges pointwise to a function  . Then
. Then  is a Picard operator. Moreover, if additionally
is a Picard operator. Moreover, if additionally  satisfies
satisfies  , then the fixed point problem is well-posed.
, then the fixed point problem is well-posed.
Proof.
Assume  has two fixed points
has two fixed points  . Then for each
. Then for each  ,
,

When  we obtain
we obtain  which is false. Hence,
which is false. Hence,  has at most one fixed point.
has at most one fixed point.
Let  . We consider
. We consider  ,
,

Because  is compact and
is compact and  -stable there exists
-stable there exists  such that
such that

For  ,
,

Letting  in the above relation yields
in the above relation yields  so
so  converges to
converges to  which will be the unique fixed point of
which will be the unique fixed point of  because
because  and
and  Thus, all the Picard iterates will converge to
Thus, all the Picard iterates will converge to  .
.
Let  be an approximate fixed point sequence and let
be an approximate fixed point sequence and let  . Then
. Then

Taking the superior limit and applying (3.2) of Proposition 3.1,

Letting  we have
we have  .
.
Theorem 3.5.
Let  be a complete
be a complete  space and let
space and let  be nonempty, bounded, closed, and convex. Let
be nonempty, bounded, closed, and convex. Let  and for
and for  , let
, let  be such that
be such that  for all
for all  . If for all
. If for all  ,
,

then  has a fixed point. Moreover,
has a fixed point. Moreover,  is closed and convex.
is closed and convex.
Proof.
The idea of the proof follows to a certain extend the proof of Theorem 5.1 in [2]. Let  . Denote
. Denote  ,
,

Since  is a nonempty, closed, and convex subset of a complete
is a nonempty, closed, and convex subset of a complete  space there exists a unique
space there exists a unique  such that
such that

For  ,
,

Let  and let
and let  denote the midpoint of the segment
denote the midpoint of the segment  . Using the (CN) inequality, we have
. Using the (CN) inequality, we have

Letting  and considering
and considering  , we have
, we have

Letting  we obtain that
we obtain that  is a Cauchy sequence which converges to
is a Cauchy sequence which converges to  . As in the proof of Theorem 3.4 we can show that
. As in the proof of Theorem 3.4 we can show that  is a fixed point for
is a fixed point for  . To prove that
. To prove that  is closed take
is closed take  a sequence of fixed points which converges to
a sequence of fixed points which converges to  . Then
. Then

which shows that  is a fixed point of
is a fixed point of  .
.
The fact that  is convex follows from the (CN) inequality. Let
is convex follows from the (CN) inequality. Let 
 and let
and let  be the midpoint of
be the midpoint of  . For
. For  we have
we have

Letting  we obtain
we obtain  . This yields
. This yields  which is a fixed point since
which is a fixed point since

Hence,  is convex.
is convex.
We conclude this section by proving a demi-closed principle similarly to [2, Proposition  ]. To this end, for
]. To this end, for  ,
,  closed and convex and
closed and convex and  , as in [2], we introduce the following notation:
, as in [2], we introduce the following notation:

where the bounded sequence  is contained in
is contained in  .
.
Theorem 3.6.
Let  be a
be a  space and let
space and let  ,
,  bounded, closed, and convex. Let
bounded, closed, and convex. Let  satisfy
satisfy  and for
and for  , let
, let  be such that
be such that  for all
for all  . Suppose that for
. Suppose that for

Let also  be an approximate fixed point sequence such that
be an approximate fixed point sequence such that  Then
Then 
 .
.
Proof.
Using (3.1) of Proposition 3.1 we obtain that for every  and every
and every  ,
,

Applying (3.2) of Proposition 3.1 for  , we have
, we have

Let  and let
and let  be the midpoint of
be the midpoint of  . As in the above proof, using the (CN) inequality we have
. As in the above proof, using the (CN) inequality we have

Since  ,
,

Letting  , we have
, we have  . This means
. This means 
 because
because

4. Generalized Strongly Asymptotic Pointwise Contractions
In this section we generalize the strongly asymptotic pointwise contraction condition, by using the diameter of the orbit. We begin with a fixed point result that holds in a complete metric space.
Theorem 4.1.
Let  be a complete metric space and let
be a complete metric space and let  be a mapping with bounded orbits that is orbitally continuous. Also, for
be a mapping with bounded orbits that is orbitally continuous. Also, for  , let
, let  for which there exists
for which there exists  such that for every
such that for every  ,
,  . If for each
. If for each

then  is a Picard operator. Moreover, if additionally
is a Picard operator. Moreover, if additionally  satisfies
satisfies  , then the fixed point problem is well-posed.
, then the fixed point problem is well-posed.
Proof.
First, suppose that  has two fixed points
has two fixed points  . Then for each
. Then for each  ,
,

Letting  we obtain that
we obtain that  which is impossible. Hence,
which is impossible. Hence,  has at most one fixed point. Let
has at most one fixed point. Let  . Notice that the sequence
. Notice that the sequence  is decreasing and bounded below by
is decreasing and bounded below by  so it converges to
so it converges to  . For
. For  we have
we have

Taking the supremum with respect to  and
and  and then letting
and then letting  we obtain
we obtain

For  ,
,

Letting  in the above relation we have
in the above relation we have  which implies that
which implies that  . This means that the sequence
. This means that the sequence  is Cauchy so it converges to a point
is Cauchy so it converges to a point  . Because
. Because  is orbitally continuous it follows that
is orbitally continuous it follows that  is a fixed point, which is unique. Therefore, all Picard iterates converge to
is a fixed point, which is unique. Therefore, all Picard iterates converge to  .
.
Next we prove that the problem is well-posed. Let  be an approximate fixed point sequence. Taking into account (3.2) applied for
be an approximate fixed point sequence. Taking into account (3.2) applied for  and (3.3) of Proposition 3.1,
and (3.3) of Proposition 3.1,

Knowing that

and taking the superior limit we obtain

If we let here  it is clear that
it is clear that  converges to
converges to  .
.
A similar result can be given in a bounded metric space where the convexity structure defined by the class of admissible subsets is compact.
Theorem 4.2.
Let  be a bounded metric space such that
be a bounded metric space such that  is compact and let
is compact and let  be an orbitally continuous mapping. Also, for
be an orbitally continuous mapping. Also, for  , let
, let  for which there exists
for which there exists  such that for every
such that for every  ,
,  . If for each
. If for each  ,
,

then  is a Picard operator. Moreover, if additionally
is a Picard operator. Moreover, if additionally  satisfies
satisfies  , then the fixed point problem is well-posed.
, then the fixed point problem is well-posed.
Proof.
Let  . Denote
. Denote  ,
,

As in the proof of Theorem 4.1 one can show that  has at most one fixed point and for each
has at most one fixed point and for each  , the sequence
, the sequence  is Cauchy. This means that
is Cauchy. This means that  for each
for each  . Because
. Because  is compact we can choose
is compact we can choose

Following the argument of [2, Theorem  ] we can show that
] we can show that  . For the sake of completeness we also include this part of the proof. The definition of
. For the sake of completeness we also include this part of the proof. The definition of  yields that for
yields that for  and every
and every  there exists
there exists  such that for any
such that for any 

Hence,  for every
for every  and so
and so

Therefore,  for each
for each  . This implies
. This implies  which holds for every
which holds for every  . Thus,
. Thus,

Now it is clear that  converges to
converges to  . Because
. Because  is orbitally continuous,
is orbitally continuous,  will be the unique fixed point and all the Picard iterates will converge to this unique fixed point.
will be the unique fixed point and all the Picard iterates will converge to this unique fixed point.
The fact that every approximate fixed point sequence  converges to
converges to  can be proved identically as in Theorem 4.1.
can be proved identically as in Theorem 4.1.
In connection with the use of the diameter of the orbit, Walter [11] obtained a fixed point theorem that may be stated as follows.
Theorem 4.3 (Walter [11]).
Let  be a complete metric space and let
be a complete metric space and let  be a mapping with bounded orbits. If there exists a continuous, increasing function
be a mapping with bounded orbits. If there exists a continuous, increasing function  for which
for which  for every
for every  and
and

then  is a Picard operator.
is a Picard operator.
We conclude this section by proving an asymptotic version of this result. In this way we extend the notion of asymptotic contraction introduced by Kirk in [12].
Theorem 4.4.
Let  be a complete metric space and let
be a complete metric space and let  be an orbitally continuous mapping with bounded orbits. Suppose there exist a continuous function
be an orbitally continuous mapping with bounded orbits. Suppose there exist a continuous function  satisfying
satisfying  for all
for all  and the functions
and the functions  such that the sequence
such that the sequence  converges pointwise to
converges pointwise to  and for each
and for each  ,
,

then  is a Picard operator. Moreover, if additionally
is a Picard operator. Moreover, if additionally  satisfies
satisfies  and
and  is continuous for each
is continuous for each  , then the fixed point problem is well-posed.
, then the fixed point problem is well-posed.
Proof.
The proof follows closely the ideas presented in the proof of Theorem 4.1.
We begin by supposing that  has two fixed points
has two fixed points  . Then for each
. Then for each  ,
,

Letting  we obtain that
we obtain that  which is impossible. Hence,
which is impossible. Hence,  has at most one fixed point.
has at most one fixed point.
Notice that for  the sequence
the sequence  is decreasing and bounded below by
is decreasing and bounded below by  so it converges to
so it converges to  . For
. For  we have
we have

Thus, 
For  ,
,

Hence,  which implies that
which implies that  and the proof may be continued as in Theorem 4.1 in order to conclude that
and the proof may be continued as in Theorem 4.1 in order to conclude that  is a Picard operator.
is a Picard operator.
Let  be the unique fixed point of
be the unique fixed point of  and let
and let  be an approximate fixed point sequence. To show that the problem is well-posed, take
be an approximate fixed point sequence. To show that the problem is well-posed, take  a subsequence of
a subsequence of  such that
such that

Because every subsequence of  is also an approximate fixed point sequence, the conclusions of Proposition 3.1 still stand for
is also an approximate fixed point sequence, the conclusions of Proposition 3.1 still stand for  . This yields
. This yields

But since

by passing to the inferior limit follows 
For 

If we let here  , we have
, we have  Passing here to the limit with respect to
Passing here to the limit with respect to  implies
implies  and this means
and this means  Because of (4.20) it follows that
Because of (4.20) it follows that  converges to
converges to  .
.
5. Some Generalized Nonexpansive Mappings in 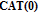 Spaces
Spaces
In this section we give fixed point results in  spaces for two classes of mappings which are more general than the nonexpansive ones.
spaces for two classes of mappings which are more general than the nonexpansive ones.
Theorem 5.1.
Let  be a bounded complete
be a bounded complete  space and let
space and let  be such that for every
be such that for every 

Then  has a fixed point. Moreover,
has a fixed point. Moreover,  is closed and convex.
is closed and convex.
Proof.
Let  . Denote
. Denote  ,
,

Since  is a complete
is a complete  space there exists a unique
space there exists a unique  such that
such that

Supposing  is not a fixed point of
is not a fixed point of  , we have
, we have

This is a contradiction and thus 
 .
.
Let  be a sequence of fixed points which converges to
be a sequence of fixed points which converges to  . Then,
. Then,

which proves that  is a fixed point of
is a fixed point of  so
so  is closed.
is closed.
Now take 
 . We show that the midpoint of
. We show that the midpoint of  denoted by
denoted by  is a fixed point of
is a fixed point of  using the (CN) inequality. More precisely we have
using the (CN) inequality. More precisely we have

Hence,  is convex.
is convex.
A simple example of a mapping which is not nonexpansive, but satisfies (5.1), is the following.
Example 5.2.
Let 

Then  is not nonexpansive but (5.1) is verified.
is not nonexpansive but (5.1) is verified.
Proof.
 is not continuous, so it cannot be nonexpansive. To show that (5.1) holds, we only consider the situation when
is not continuous, so it cannot be nonexpansive. To show that (5.1) holds, we only consider the situation when  and
and  because in all other the condition is clearly satisfied. Then
because in all other the condition is clearly satisfied. Then  We can easily observe that
We can easily observe that

If  then
then  Otherwise,
Otherwise,  In this way we have shown that (5.1) is accomplished.
In this way we have shown that (5.1) is accomplished.
Theorem 5.3.
Let  be a bounded complete
be a bounded complete  space and let
space and let  be such that for every
be such that for every  ,
,


Then  has a fixed point. Moreover,
has a fixed point. Moreover,  is closed and convex.
is closed and convex.
Proof.
Let  . Denote
. Denote  ,
,

Since  is a complete
is a complete  space there exists a unique
space there exists a unique  such that
such that

Let  This limit exists since the sequence is decreasing and bounded below by
This limit exists since the sequence is decreasing and bounded below by  .
.
Suppose  is not a fixed point of
is not a fixed point of  . Then
. Then

This means that


Inductively, it follows that for  ,
,

Let  and let
and let  . Obviously,
. Obviously,

since 
Because of (5.9) we have

Since  , it is clear that
, it is clear that 
Hence,

Let 
Then,

Taking into account (5.14),  Now,
Now,

which is a contradiction. Hence,  .
.
The fact that  is closed and convex follows as in the previous proof.
is closed and convex follows as in the previous proof.
Remark 5.4.
It is clear that nonexpansive mappings and mappings for which (5.1) holds satisfy (5.9) and (5.10). However, there are mappings which satisfy these two conditions without verifying (5.1) as the following example shows.
Example 5.5.
The set  with the usual metric is a
with the usual metric is a  space. Let us take
space. Let us take  ,
,

Then  does not satisfy (5.1) but conditions (5.9), (5.10) hold.
does not satisfy (5.1) but conditions (5.9), (5.10) hold.
Proof.
To prove that  does not verify (5.1) we take
does not verify (5.1) we take  and
and  . Then
. Then  However,
However,

Next we show that (5.9) and (5.10) hold. We only need to consider the case when  and
and  because in all the other situations this is evident. Then
because in all the other situations this is evident. Then  Since
Since

relation (5.9) is satisfied.
Also,

Since  we obtain
we obtain  Hence, relation (5.10) is also accomplished.
Hence, relation (5.10) is also accomplished.
Remark 5.6.
If we replace condition (5.9) of Theorem 5.3 with

where  , then we may conclude that
, then we may conclude that  has s unique fixed point.
has s unique fixed point.
It is also clear that a pointwise contraction satisfies these conditions so we can apply this result to prove that it has a unique fixed point.
We next prove a demi-closed principle. We will use the notations introduced at the end of Section 3.
Theorem 5.7.
Let  be a
be a  space,
space,  ,
,  bounded, closed, and convex. Let
bounded, closed, and convex. Let  be a mapping that safisfies
be a mapping that safisfies  and (5.9) for each
and (5.9) for each  and let
and let  be an approximate fixed point sequence such that
be an approximate fixed point sequence such that  Then
Then 
 .
.
Proof.
Using (3.1) of Proposition 3.1 we have  Applying (3.2) and (3.3) of Proposition 3.1 for
Applying (3.2) and (3.3) of Proposition 3.1 for  ,
,

Then,

Let  denote the midpoint of
denote the midpoint of  . The (CN) inequality yields
. The (CN) inequality yields

Taking the superior limit, we have

But since  ,
,

Hence, 
 .
.
We conclude this paper with the following remarks.
Remark 5.8.
All the above results obtained in the context of  spaces also hold in the more general setting used in [4] of uniformly convex metric spaces with monotone modulus of convexity.
spaces also hold in the more general setting used in [4] of uniformly convex metric spaces with monotone modulus of convexity.
Remark 5.9.
In a similar way as for nonexpansive mappings, one can develop a theory for the classes of mappings introduced in this section. An interesting idea would be to study the approximate fixed point property of such mappings. A nice synthesis in the case of nonexpansive mappings can be found in the recent paper of Kirk [13].
References
Kirk WA, Xu H-K: Asymptotic pointwise contractions. Nonlinear Analysis: Theory, Methods & Applications 2008,69(12):4706–4712. 10.1016/j.na.2007.11.023
Hussain N, Khamsi MA: On asymptotic pointwise contractions in metric spaces. Nonlinear Analysis: Theory, Methods & Applications 2009,71(10):4423–4429. 10.1016/j.na.2009.02.126
Espínola R, Hussain N: Common fixed points for multimaps in metric spaces. Fixed Point Theory and Applications 2010, Article ID 204981 2010:-14 Pages.
Espínola R, Fernández-León A, Piątek B: Fixed points of single- and set-valued mappings in uniformly convex metric spaces with no metric convexity. Fixed Point Theory and Applications 2010, Article ID 204981 2010:-16 .
Reich S, Zaslavski AJ: Well-posedness of fixed point problems. Far East Journal of Mathematical Sciences, Special Volume Part III 2001, 393–401.
Rus IA: Picard operators and well-posedness of fixed point problems. Studia Universitatis Babeş-Bolyai Mathematica 2007,52(3):147–156.
Bruhat F, Tits J: Groupes réductifs sur un corps locall: I. Données radicielles valuées. Institut des Hautes Études Scientifiques. Publications Mathématiques 1972, (41):5–251.
Dhompongsa S, Kirk WA, Sims B: Fixed points of uniformly lipschitzian mappings. Nonlinear Analysis: Theory, Methods & Applications 2006,65(4):762–772. 10.1016/j.na.2005.09.044
Kirk WA: Geodesic geometry and fixed point theory. In Seminar of Mathematical Analysis (Malaga/Seville, 2002/2003). Volume 64. Edited by: Girela D, López G, Villa R. Universities of Malaga and Seville, Sevilla, Spain; 2003:195–225.
Kirk WA: Geodesic geometry and fixed point theory II. In Fixed Point Theory and Applications. Edited by: García-Falset J, Llorens-Fuster E, Sims B. Yokohama, Yokohama, Japan; 2004:113–142.
Walter W: Remarks on a paper by F. Browder about contraction. Nonlinear Analysis: Theory, Methods & Applications 1981,5(1):21–25. 10.1016/0362-546X(81)90066-3
Kirk WA: Fixed points of asymptotic contractions. Journal of Mathematical Analysis and Applications 2003,277(2):645–650. 10.1016/S0022-247X(02)00612-1
Kirk WA: Approximate fixed points of nonexpansive maps. Fixed Point Theory 2009,10(2):275–288.
Acknowledgment
The author wishes to thank the financial support provided from programs cofinanced by The Sectoral Operational Programme Human Resources Development, Contract POS DRU 6 1.5
1.5 S
S 3—"Doctoral studies: through science towards society."
3—"Doctoral studies: through science towards society."
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License (https://creativecommons.org/licenses/by/2.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
About this article
Cite this article
Nicolae, A. Generalized Asymptotic Pointwise Contractions and Nonexpansive Mappings Involving Orbits. Fixed Point Theory Appl 2010, 458265 (2009). https://doi.org/10.1155/2010/458265
Received:
Accepted:
Published:
DOI: https://doi.org/10.1155/2010/458265
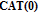 Spaces
Spaces