- Research Article
- Open access
- Published:
Results on the Existence and Convergence of Best Proximity Points
Fixed Point Theory and Applications volume 2010, Article number: 386037 (2010)
Abstract
We first consider a cyclic  -contraction map on a reflexive Banach space
-contraction map on a reflexive Banach space  and provide a positive answer to a question raised by Al-Thagafi and Shahzad on the existence of best proximity points for cyclic
and provide a positive answer to a question raised by Al-Thagafi and Shahzad on the existence of best proximity points for cyclic  -contraction maps in reflexive Banach spaces in one of their works (2009). In the second part of the paper, we will discuss the existence of best proximity points in the framework of more general metric spaces. We obtain some new results on the existence of best proximity points in hyperconvex metric spaces as well as in ultrametric spaces.
-contraction maps in reflexive Banach spaces in one of their works (2009). In the second part of the paper, we will discuss the existence of best proximity points in the framework of more general metric spaces. We obtain some new results on the existence of best proximity points in hyperconvex metric spaces as well as in ultrametric spaces.
1. Introduction
Let  be a metric space, and let
be a metric space, and let  be two subsets of
be two subsets of  . A mapping
. A mapping  is said to be cyclic provided that
is said to be cyclic provided that  and
and  . In [1] Kirk et al. proved the following interesting extension of the Banach contraction principle:
. In [1] Kirk et al. proved the following interesting extension of the Banach contraction principle:
Theorem 1.1 (see [1]).
Let  and
and  be two nonempty closed subsets of a complete metric space
be two nonempty closed subsets of a complete metric space  . Suppose that
. Suppose that  is a cyclic map such that
is a cyclic map such that

for some  and for all
and for all  . Then
. Then  has a unique fixed point in
has a unique fixed point in  .
.
Later on, Eldred and Veeramani [2] considered the class of cyclic contractions.
Definition 1.2 (see [2]).
Let  and
and  be two nonempty subsets of a metric space
be two nonempty subsets of a metric space  , and let
, and let  , and
, and  . We say that
. We say that  is a cyclic contraction if
is a cyclic contraction if

for some  and for all
and for all  , where
, where

We recall that a point  is said to be a best proximity point for
is said to be a best proximity point for  provided that
provided that  .
.
In the case that  is a uniformly convex Banach space, Eldred and Veeramani established the following theorem.
is a uniformly convex Banach space, Eldred and Veeramani established the following theorem.
Theorem 1.3 (see [2]).
Let  and
and  be two nonempty closed convex subsets of a uniformly convex Banach space
be two nonempty closed convex subsets of a uniformly convex Banach space  , and let
, and let  be a cyclic contraction map. For
be a cyclic contraction map. For  , define
, define  for each
for each  . Then there exists a unique
. Then there exists a unique  such that
such that  and
and  .
.
In 2009, Al-Thagafi and Shahzad introduced a new class of mappings, namely, the class of cyclic  -contraction maps. This new class contains the class of cyclic contraction maps.
-contraction maps. This new class contains the class of cyclic contraction maps.
Definition 1.4 (see [3]).
Let  and
and  be two nonempty subsets of a metric space
be two nonempty subsets of a metric space  and let
and let  be a mapping such that
be a mapping such that  and
and  .
.  is said to be a cyclic
is said to be a cyclic  -contraction map if there exists a strictly increasing function
-contraction map if there exists a strictly increasing function  such that
such that

for all  and
and  .
.
In [3] the authors were able to establish some existence and convergence results for these mappings. Moreover, they proved the existence of a best proximity point for a cyclic contraction map in a reflexive Banach space  (see [3, Theorems
(see [3, Theorems  ,
,  ]). In this way they answered a question raised by Eldred and Veeramani in the affirmative. We recall that Theorem 1.3 above was proved in the setting of a uniformly convex Banach space. The authors of [3] then asked if the result stands true if we assume that
]). In this way they answered a question raised by Eldred and Veeramani in the affirmative. We recall that Theorem 1.3 above was proved in the setting of a uniformly convex Banach space. The authors of [3] then asked if the result stands true if we assume that  is a reflexive Banach space, rather than being uniformly convex.
is a reflexive Banach space, rather than being uniformly convex.
Al-Thagafi and N. Shahzad then stated it was interesting to ask whether Theorems  and
and  (resp., Theorems
(resp., Theorems  and
and  ) held true for cyclic
) held true for cyclic  -contraction maps when the Banach space in question is only reflexive (resp., reflexive and strictly convex).
-contraction maps when the Banach space in question is only reflexive (resp., reflexive and strictly convex).
In this paper we first take up these questions. It turns out that under some conditions the answer is positive. In the last section we study the existence of best proximity points in spherically complete ultrametric spaces, as well as in hyperconvex metric spaces. More precisely, we will see that best proximity points exist for cyclic  -contraction maps on hyperconvex metric spaces. We will also provide an existence theorem for a cyclic map which satisfies some contractive condition on an ultrametric space.
-contraction maps on hyperconvex metric spaces. We will also provide an existence theorem for a cyclic map which satisfies some contractive condition on an ultrametric space.
2. Cyclic  -Contraction Maps
-Contraction Maps
In this section we first provide a positive answer to the question raised by the authors of [3]. Then we present some consequences and applications. Among other things, is a common fixed point theorem for two maps. We will begin with the following lemma.
Lemma 2.1 (see [3, Lemma  ]).
]).
Let  and
and  be two nonempty subsets of a metric space
be two nonempty subsets of a metric space  and let
and let  be a cyclic
be a cyclic  -contraction map. For
-contraction map. For  , define
, define  for each
for each  . Then one has
. Then one has
(a) for all
for all  and
and  ,
,
(b) for all
for all  and
and  ,
,
(c) for all
for all  .
.
Now we state and prove the following lemma which is key to the proof of the main result of this section.
Lemma 2.2.
Let  and
and  be two nonempty subsets of a metric space
be two nonempty subsets of a metric space  , and let
, and let  be a cyclic
be a cyclic  -contraction map. For
-contraction map. For  , define
, define  for each
for each  . Then the sequences
. Then the sequences  , and
, and  are bounded if either of the following conditions holds:
are bounded if either of the following conditions holds:
(i) ,
,
(ii)
Proof.
We first show that the sequence  is bounded. Suppose the contrary. Then for every positive integer
is bounded. Suppose the contrary. Then for every positive integer  , there exists
, there exists  such that
such that

We note that

According to Lemma 2.1,  is nonexpansive, so that (by the property of
is nonexpansive, so that (by the property of  )
)

Therefore

But since  is increasing, it follows that
is increasing, it follows that

Thus

This implies that for every positive integer  we have
we have

contradicting the hypothesis that  .
.
We now assume that condition (ii) holds. It follows from (2.7) that

Since (2.8) holds for all  , we conclude that
, we conclude that

for all  Letting now
Letting now  and using Theorem
and using Theorem  of [3] we conclude that
of [3] we conclude that

which contradicts the fact that  is strictly increasing.
is strictly increasing.
This arguments show that the sequence  is bounded. But since
is bounded. But since

and that both terms on the right-hand side are bounded, we conclude that  is bounded.
is bounded.
Similarly, by considering the sequence  we can prove that the sequence
we can prove that the sequence  is bounded.
is bounded.
We now come to the first main result of this paper generalizing Theorem  of [3] to cyclic
of [3] to cyclic  -contraction maps.
-contraction maps.
Theorem 2.3.
Let  and
and  be two nonempty weakly closed subsets of a reflexive Banach space
be two nonempty weakly closed subsets of a reflexive Banach space  and let
and let  be a cyclic
be a cyclic  -contraction map satisfying either of the following:
-contraction map satisfying either of the following:
(i) ,
,
(ii) .
.
Then there exists  such that
such that  .
.
Proof.
Let  be arbitrarily chosen. We define
be arbitrarily chosen. We define  . It follows from Lemma 2.2 that the sequences
. It follows from Lemma 2.2 that the sequences  and
and  are bounded in
are bounded in  and in
and in  , respectively. Since
, respectively. Since  is reflexive, every bounded sequence in
is reflexive, every bounded sequence in  has a weakly convergent subsequence. Assume that
has a weakly convergent subsequence. Assume that  weakly. Since
weakly. Since  is weakly closed,
is weakly closed,  . Similarly, we may assume that there is a
. Similarly, we may assume that there is a  such that
such that  , weakly. Therefore
, weakly. Therefore  , weakly. But according to a well-known fact in basic functional analysis, we have
, weakly. But according to a well-known fact in basic functional analysis, we have

from which it follows that  .
.
Remark 2.4.
If we assume that the function  satisfies either of the conditions (i) or (ii) of Lemma 2.2, then all three theorems (Theorems
satisfies either of the conditions (i) or (ii) of Lemma 2.2, then all three theorems (Theorems  , 11, and 12 of [3] can be generalized to cyclic
, 11, and 12 of [3] can be generalized to cyclic  -contraction maps. We omit the details.
-contraction maps. We omit the details.
The next theorem generalizes Theorem 1.1 to reflexive Banach spaces. Note that if  and
and  for some fixed
for some fixed  , then
, then  will be a cyclic contraction map, because for all
will be a cyclic contraction map, because for all  and all
and all  we have
we have

Theorem 2.5.
Let  and
and  be two nonempty subsets of a reflexive Banach space
be two nonempty subsets of a reflexive Banach space  such that
such that  is weakly closed. Let
is weakly closed. Let  be a cyclic
be a cyclic  -contraction map which is weakly continuous on
-contraction map which is weakly continuous on  . For
. For  , define
, define  for each
for each  . If
. If  then
then  has a unique fixed point
has a unique fixed point  and
and  .
.
Proof.
Since  is cyclic
is cyclic  -contraction, and
-contraction, and  , it follows from Lemma 2.2 that
, it follows from Lemma 2.2 that  is bounded in
is bounded in  . Therefore we can find a weak convergent subsequence, say
. Therefore we can find a weak convergent subsequence, say  , to a point
, to a point  . On the other hand,
. On the other hand,  is weakly continuous, so that
is weakly continuous, so that  weakly. It follows that
weakly. It follows that

As in the proof of Theorem 2.3 we conclude that  . The proof of uniqueness part is a verbatim repetition of the proof of Theorem
. The proof of uniqueness part is a verbatim repetition of the proof of Theorem  in [3]. We omit the details.
in [3]. We omit the details.
As an application of Theorem 2.5, we will prove a theorem on the existence and approximation of common fixed points for two maps.
Theorem 2.6.
Let  be a nonempty subset of a reflexive Banach space
be a nonempty subset of a reflexive Banach space  and
and  be two maps such that
be two maps such that  is weakly closed in
is weakly closed in  and
and  . Let
. Let  be a cyclic
be a cyclic  -contraction map that satisfies this property that if there exist
-contraction map that satisfies this property that if there exist  such that
such that  , then
, then  commutes with
commutes with  in
in  . Then
. Then  have a common fixed point in
have a common fixed point in  . Moreover, if
. Moreover, if  ,
,  and
and  for each
for each  then the sequence
then the sequence  converges to a common fixed point of
converges to a common fixed point of  .
.
Proof.
By Theorem 2.5 there exists a unique  such that
such that  . Since
. Since  , there exists
, there exists  such that
such that  so that
so that  . Also there exists
. Also there exists  such that
such that  , so that
, so that  . Now we have
. Now we have

That is,  is a fixed point for
is a fixed point for  . Since the fixed point of
. Since the fixed point of  is unique, we must have
is unique, we must have  . Therefore
. Therefore  is a fixed point of
is a fixed point of  . Similarly we can show that
. Similarly we can show that  is a fixed point of
is a fixed point of  . Consequently
. Consequently  is a common fixed point for
is a common fixed point for  . According to Theorem 2.5 the sequence
. According to Theorem 2.5 the sequence  converges to
converges to  .
.
Example 2.7.
Let  and
and  . Let
. Let  and define
and define  with
with  and
and  . Also consider
. Also consider  by
by  . Then
. Then  is cyclic contraction and satisfies the conditions of Theorem 2.6. Therefore
is cyclic contraction and satisfies the conditions of Theorem 2.6. Therefore  have a common fixed point. It is clear that this common fixed point is
have a common fixed point. It is clear that this common fixed point is  .
.
3. Cyclic 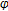 -Contraction Maps in Metric Spaces
-Contraction Maps in Metric Spaces
In this section we discuss the existence of best proximity points for cyclic  -contraction maps in metric spaces. Indeed we prove two existence theorems on best proximity points in hyperconvex spaces, as well as in ultrametric spaces.
-contraction maps in metric spaces. Indeed we prove two existence theorems on best proximity points in hyperconvex spaces, as well as in ultrametric spaces.
Lemma 3.1.
Let  be two nonempty subsets of a metric space
be two nonempty subsets of a metric space  , and Let
, and Let  be a cyclic
be a cyclic  -contraction map. If there exists
-contraction map. If there exists  such that
such that  , then
, then  has a best proximity point.
has a best proximity point.
Proof.
Since  , then
, then  is fixed point for
is fixed point for  Therefore we have
Therefore we have

Thus  . Since
. Since  is strictly increasing, we conclude that
is strictly increasing, we conclude that

In the following definition we will use the notation  for the Kuratowski measure of noncompactness of a given set
for the Kuratowski measure of noncompactness of a given set  . For more information see the book written by Khamsi and Kirk [4].
. For more information see the book written by Khamsi and Kirk [4].
Definition 3.2.
Let  be a subset of a metric space
be a subset of a metric space  . A mapping
. A mapping  is said to be condensing if
is said to be condensing if  is bounded and continuous, moreover
is bounded and continuous, moreover  , for every bounded subset
, for every bounded subset  of
of  for which
for which  .
.
Definition 3.3 (see [4]).
A metric space  is called hyperconvex if for any indexed class of closed balls
is called hyperconvex if for any indexed class of closed balls  , of
, of  which satisfy
which satisfy

it is necessarily the case that 
We recall that for a given set  , the notation
, the notation  denotes the family of all admissible subsets of
denotes the family of all admissible subsets of  , that is, the family of subsets of
, that is, the family of subsets of  that can be written as the intersection of a family of closed balls centered at points of
that can be written as the intersection of a family of closed balls centered at points of  . For further information on the subject we refer the reader to [4]. We now state and prove the first main result of this section.
. For further information on the subject we refer the reader to [4]. We now state and prove the first main result of this section.
Theorem 3.4.
Let  be a hyperconvex metric space, and
be a hyperconvex metric space, and  be two nonempty subsets of
be two nonempty subsets of  such that
such that  . Suppose
. Suppose  is a cyclic
is a cyclic  -contraction map. Put
-contraction map. Put  and
and  . If
. If  is a condensing map then
is a condensing map then  has a best proximity point.
has a best proximity point.
Proof.
Since  is a hyperconvex metric space, and since
is a hyperconvex metric space, and since  , it follows from Proposition
, it follows from Proposition  of [5] that
of [5] that  is a hyperconvex metric space too. On the other hand,
is a hyperconvex metric space too. On the other hand,  is a condensing map, thus by Theorem
is a condensing map, thus by Theorem  of [5],
of [5],  or
or  has a fixed point. It now follows from Lemma 3.1 that
has a fixed point. It now follows from Lemma 3.1 that  has a best proximity point.
has a best proximity point.
Definition 3.5.
A metric space  is an ultrametric space if, in addition to the usual metric axioms, the following property holds for each
is an ultrametric space if, in addition to the usual metric axioms, the following property holds for each  :
:

For example if  is a discrete metric space then
is a discrete metric space then  is an ultrametric space. Ultrametric spaces arise in the study of non-Archimedean analysis, and in particular in the study of Banach space over non-Archimedean valuation fields (see [4]).
is an ultrametric space. Ultrametric spaces arise in the study of non-Archimedean analysis, and in particular in the study of Banach space over non-Archimedean valuation fields (see [4]).
Remark 3.6.
It is immediate from Definition 3.5 that if  and
and  are two closed balls in an ultrametric space, with
are two closed balls in an ultrametric space, with  , then either
, then either  or
or  In particular if
In particular if  , then
, then 
Definition 3.7.
An ultrametric space  is said to be spherically complete if every chain of closed balls in
is said to be spherically complete if every chain of closed balls in  has nonempty intersection.
has nonempty intersection.
As a consequence of Remark 3.6, the admissible sets  of
of  coincide with the closed balls of
coincide with the closed balls of  . Here we state and prove the second main result of this section.
. Here we state and prove the second main result of this section.
Theorem 3.8.
Suppose  is a spherically complete ultrametric space and
is a spherically complete ultrametric space and  are two nonempty subsets of
are two nonempty subsets of  such that
such that  . Let
. Let  be a cyclic map which satisfies the following condition:
be a cyclic map which satisfies the following condition:

for each  and for some
and for some  Then
Then  has a best proximity point.
has a best proximity point.
Proof.
Let  and define
and define  for
for  . Put
. Put  By Theorem
By Theorem  of [6],
of [6],  Now if there exits
Now if there exits  such that
such that  , then
, then

Therefore  . This argument shows that
. This argument shows that  has a best proximity point.
has a best proximity point.
Now let for all  , we have
, we have  Thus
Thus

Then  (all balls are assumed to be closed). Now by Remark 3.6 we have
(all balls are assumed to be closed). Now by Remark 3.6 we have

This shows that  is a descending chain of closed balls in
is a descending chain of closed balls in  ; in particular, each two members of this chain intersect. It is rather obvious that each member of this chain also intersects
; in particular, each two members of this chain intersect. It is rather obvious that each member of this chain also intersects  (because
(because  ). Since
). Since  and
and  is a spherically complete ultrametric space, then
is a spherically complete ultrametric space, then  itself is a closed ball (see [4, page 114]). Now each two elements of the family consisting of
itself is a closed ball (see [4, page 114]). Now each two elements of the family consisting of  and
and  intersects. Therefore if we set
intersects. Therefore if we set  , according to [4, page 115], there exists a point
, according to [4, page 115], there exists a point  which belongs to
which belongs to  as well. Therefore
as well. Therefore

But for the second term we have

because

It now follows that

Since the above relation holds for all  then we have
then we have

Therefore  , which means that
, which means that  has a best proximity point.
has a best proximity point.
In the following example we will see that the condition that  is spherically complete is necessary.
is spherically complete is necessary.
Example 3.9.
Let  and define a metric
and define a metric  on
on  by
by

It is clear that  is a complete ultrametric space (see [5]). Set
is a complete ultrametric space (see [5]). Set

and define the mapping  by
by  It is easy to see that
It is easy to see that  is cyclic and
is cyclic and  It is not difficult to see that
It is not difficult to see that  satisfies the relation (3.5) of the previous theorem for
satisfies the relation (3.5) of the previous theorem for  but
but  has no best proximity point. To see this, assume that
has no best proximity point. To see this, assume that

for some  . Thus
. Thus  which is impossible. We claim that the ultrametric space
which is impossible. We claim that the ultrametric space  is not spherically complete.
is not spherically complete.
Consider the family of closed balls  in
in  Since
Since

it follows from Remark 3.6 that

Therefore this family is a chain of closed balls in  Now let
Now let

for some  This implies that for all
This implies that for all  we have
we have

which is a contradiction.
References
Kirk WA, Srinivasan PS, Veeramani P: Fixed points for mappings satisfying cyclical contractive conditions. Fixed Point Theory 2003,4(1):79–89.
Eldred AA, Veeramani P: Existence and convergence of best proximity points. Journal of Mathematical Analysis and Applications 2006,323(2):1001–1006. 10.1016/j.jmaa.2005.10.081
Al-Thagafi MA, Shahzad N: Convergence and existence results for best proximity points. Nonlinear Analysis: Theory, Methods & Applications 2009,70(10):3665–3671. 10.1016/j.na.2008.07.022
Khamsi MA, Kirk WA: An Introduction to Metric Spaces and Fixed Point Theory, Pure and Applied Mathematics. Wiley-Interscience, New York, NY, USA; 2001:x+302.
Jachymski J: A note on a paper of I. D. Aradelović on asymptotic contractions. Journal of Mathematical Analysis and Applications 2009,358(2):491–492. 10.1016/j.jmaa.2009.05.012
Suzuki T, Kikkawa M, Vetro C: The existence of best proximity points in metric spaces with the property UC. Nonlinear Analysis: Theory, Methods & Applications 2009,71(7–8):2918–2926. 10.1016/j.na.2009.01.173
Rezapour Sh, Derafshpour M, Shahzad N: Best proximity points of cyclic -contractions on reflexive Banach spaces. Fixed Point Theory and Applications 2010, Article ID 946178 2010:-7.
Acknowledgment
After the appearance of this paper on the current journal home page, the authors have been informed by Nasser Shahzad and Shahram Rezapour that they already published paper [7], answering a question raised by the authors of [3]. The current authors would like to thank them for this piece of information.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License (https://creativecommons.org/licenses/by/2.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
About this article
Cite this article
Abkar, A., Gabeleh, M. Results on the Existence and Convergence of Best Proximity Points. Fixed Point Theory Appl 2010, 386037 (2010). https://doi.org/10.1155/2010/386037
Received:
Accepted:
Published:
DOI: https://doi.org/10.1155/2010/386037
 -Contraction Maps
-Contraction Maps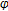 -Contraction Maps in Metric Spaces
-Contraction Maps in Metric Spaces